Advertisements
Advertisements
प्रश्न
In ΔABC, AB = AC, BM and Cn are perpendiculars on AC and AB respectively. Prove that BM = CN.
उत्तर
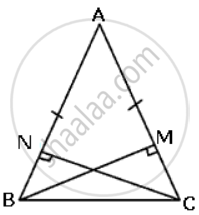
In ΔBNC and ΔCMB
∠BNC = ∠CMB = 90°
∠NBC = ∠MCB ...(AB = AC)
BC = BC
Therefore, ΔBNC ≅ ΔCMB ...(AAS criteria)
Hence, BM = CN.
APPEARS IN
संबंधित प्रश्न
If ΔDEF ≅ ΔBCA, write the part(s) of ΔBCA that correspond to `bar(EF)`
In a squared sheet, draw two triangles of equal areas such that
The triangles are congruent.
What can you say about their perimeters?
Prove that the sum of three altitudes of a triangle is less than the sum of its sides.
ΔPQR and ΔABC is not congruent to ΔRPQ, then which of the following is not true:
In the following example, a pair of triangles is shown. Equal parts of triangle in each pair are marked with the same sign. Observe the figure and state the test by which the triangle in each pair are congruent.
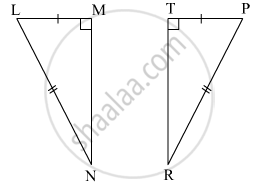
By ______ test
ΔLMN ≅ ΔPTR
In the given figure, prove that:
(i) ∆ ACB ≅ ∆ ECD
(ii) AB = ED

In ΔABC, AB = AC and the bisectors of angles B and C intersect at point O.Prove that BO = CO and the ray AO is the bisector of angle BAC.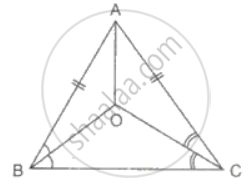
ΔABC is an isosceles triangle with AB = AC. GB and HC ARE perpendiculars drawn on BC.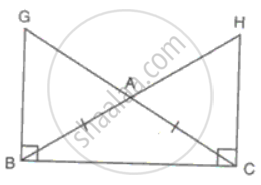
Prove that
(i) BG = CH
(ii) AG = AH
Is it possible to construct a triangle with lengths of its sides as 4 cm, 3 cm and 7 cm? Give reason for your answer.
Without drawing the triangles write all six pairs of equal measures in the following pairs of congruent triangles.
∆STU ≅ ∆DEF
