Advertisements
Advertisements
प्रश्न
The acceleration due to gravity on the surface of the moon is 1.7 m/s2. What is the time period of a simple pendulum on the surface of the moon if its time period on the surface of the earth is 3.5 s? (g on the surface of earth = 9.8 m/s2)
उत्तर
Given: gm = 1.7 m/s2, gE = 9.8 m/s2, TE = 3.5 s
To find: Time period on the surface of the moon (Tm)
Formula: `"T" = 2pisqrt("L"/"g")`
Calculation:
From formula,
`"T"_"m"/"T"_"E" = sqrt("g"_"E"/"g"_"m")`
∴ Tm = `sqrt(9.8/1.7) xx 3.5`
= 8.40 s
The time period of a simple pendulum on the surface of the moon is 8.40 s.
संबंधित प्रश्न
Potential energy of a particle performing linear S.H.M. is 0.1 π2x2 joule. If the mass of the particle is 20 g, find the frequency of S.H.M.
Two S.H.M.’s have zero phase difference and equal amplitudes A. The resultant amplitude on their composition will be ______
What is the amplitude of S.H.M.
A particle performing S.H.M. has velocities of 8 cm/s and 6 cm/s at displacements of 3 cm and 4 cm respectively. Calculate the amplitude and period of S.H.M.
Obtain an expression for the resultant amplitude of, the composition of two S.H.M.’s having the same period along the same path.
In amplitude modulation,
The total energy of the body executing S.H.M. is E. The kinetic energy of the body, when the displacement is half of the amplitude is ______.
When a mass is hung from a light spring, the spring extends by 10 cm. If the mass is pulled down and let go, it executes S.H.M. with a time period (g = 10 m/s2) ____________.
A particle is executing S.H.M. with amplitude of 4 cm and time period 12 s. The time taken by the particle in going from its mean position to a position of displacement equal to 2 cm is T1 The time taken from this displaced position of 2 cm to reach the extreme position is T2. T1/ T2 will be____________.
The amplitude of sound is doubled and the frequency is reduced to one fourth. The intensity of sound at the same point will be ____________.
A body of mass 1 kg is suspended from a spring of negligible mass. Another body of mass 500 g moving vertically upwards hits the suspended body with a velocity 3 ms-1 and gets embedded in it. If the frequency of oscillation of the system of the two bodies after collision `10/pi` Hz, the amplitude of motion and the spring constant are respectively ____________.
A horizontal spring executes S.H.M. with amplitude 'A1', when mass 'm1' is attached to it, When it passes through mean position another mass 'm2' is placed on it. Both masses move together with amplitude 'A2'. Therefore A2 : A1 is ______
A mass M attached to a horizontal spring executes S.H.M. of amplitude A1. When the mass M passes through its mean position, then a smaller mass m is placed over it and both of them move together with amplitude A2. The ratio of `(A_1/A_2)` is ______
Two trains are moving towards each other with speeds of 20m/s and 15 m/s relative to the ground. The first train sounds a whistle of frequency 600 Hz. The frequency of the whistle heard by a passenger in the second train before the train meets, is ______. (the speed of sound in air is 340 m/s)
A mass is suspended from a vertical spring which is executing S.H.M. of frequency 5 Hz. The spring is unstretched at the highest point of oscillation. Maximum speed of the mass is ______. [acceleration due to gravity g = 10 m/s2]
A mass m1 connected to a horizontal spring performs SHM with amplitude A. While mass m1 is passing through mean position, another mass m2 is placed on it so that both the masses move together with amplitude A1. The ratio of `"A"_1/"A"` is ______. (m2 < m1)
A sinusoidal wave travelling in the same direction have amplitudes of 3 cm and 4 cm and difference in phase by `pi/2`. The resultant amplitude of the superimposed wave is ______.
The motion of a particle varies with time according to the relation y = a sin ω t + a cos ω t. Then, ______.
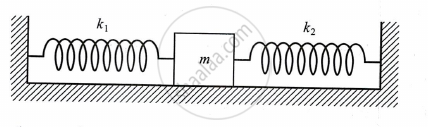
A block of mass m, connected to two springs of spring constants k1 and k2 as shown, oscillates on a smooth horizontal surface. What is the effective spring constant of the oscillation?
A particle performs linear SHM with amplitude A and frequency n. Its speed midway between an extreme position and equilibrium position is ______.
