Advertisements
Advertisements
प्रश्न
The area between x-axis and curve y = cos x when 0 ≤ x ≤ 2 π is ___________ .
पर्याय
0
2
3
4
उत्तर
` 4`
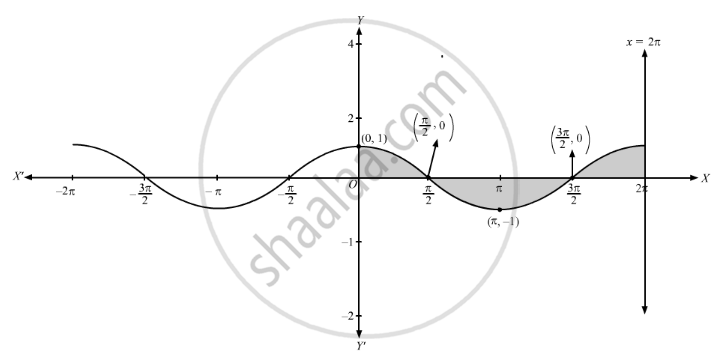
Required shaded area,
\[A = \int_0^\frac{\pi}{2} \cos x dx + \int_\frac{\pi}{2}^\frac{3\pi}{2} \left( - \cos x \right)dx + \int_{3\frac{\pi}{2}}^{2\pi} \cos x dx\]
\[ = \int_0^\frac{\pi}{2} \cos x dx - \int_\frac{\pi}{2}^\frac{3\pi}{2} \cos x dx + \int_{3\frac{\pi}{2}}^{2\pi} \cos x dx\]
\[ = \left[ \sin x \right]_0^\frac{\pi}{2} - \left[ \sin x \right]_\frac{\pi}{2}^\frac{3\pi}{2} + \left[ \sin x \right]_{3\frac{\pi}{2}}^{2\pi} \]
\[ = \left[ \sin x \right]_0^\frac{\pi}{2} - \left[ \sin x \right]_\frac{\pi}{2}^\frac{3\pi}{2} + \left[ \sin x \right]_{3\frac{\pi}{2}}^{2\pi} \]
\[ = \left( 1 - 0 \right) - \left( - 1 - 1 \right) + \left[ 0 - \left( - 1 \right) \right]\]
\[ = 1 + 2 + 1\]
\[ = 4\text{ sq units }\]
APPEARS IN
संबंधित प्रश्न
Using integration, find the area of the region bounded by the triangle whose vertices are (−1, 2), (1, 5) and (3, 4).
Find the area of the region lying between the parabolas y2 = 4ax and x2 = 4ay.
Find the area bounded by curves (x – 1)2 + y2 = 1 and x2 + y 2 = 1
Find the area of the region bounded by the curves y = x2 + 2, y = x, x = 0 and x = 3
Using integration find the area of the triangular region whose sides have the equations y = 2x +1, y = 3x + 1 and x = 4.
Smaller area enclosed by the circle x2 + y2 = 4 and the line x + y = 2 is
A. 2 (π – 2)
B. π – 2
C. 2π – 1
D. 2 (π + 2)
Area lying between the curve y2 = 4x and y = 2x is
A. 2/3
B. 1/3
C. 1/4
D. 3/4
Using the method of integration find the area bounded by the curve |x| + |y| = 1 .
[Hint: The required region is bounded by lines x + y = 1, x– y = 1, – x + y = 1 and
– x – y = 1].
Choose the correct answer The area of the circle x2 + y2 = 16 exterior to the parabola y2 = 6x is
A. `4/3 (4pi - sqrt3)`
B. `4/3 (4pi + sqrt3)`
C. `4/3 (8pi - sqrt3)`
D.`4/3 (4pi + sqrt3)`
The area bounded by the y-axis, y = cos x and y = sin x when 0 <= x <= `pi/2`
(A) 2 ( 2 −1)
(B) `sqrt2 -1`
(C) `sqrt2 + 1`
D. `sqrt2`
The area enclosed between the curves y = loge (x + e), x = loge \[\left( \frac{1}{y} \right)\] and the x-axis is _______ .
Area lying between the curves y2 = 4x and y = 2x is
Find the area of the ellipse `x^2/1 + y^2/4` = 1, in first quadrant
Find the area of sector bounded by the circle x2 + y2 = 25, in the first quadrant−
Find the area of the ellipse `x^2/36 + y^2/64` = 1, using integration
Find the area of the region lying between the parabolas 4y2 = 9x and 3x2 = 16y
Find the area of the region included between y = x2 + 5 and the line y = x + 7
Find the area enclosed between the circle x2 + y2 = 9, along X-axis and the line x = y, lying in the first quadrant
Find the area of a minor segment of the circle x2 + y2 = a2 cut off by the line x = `"a"/2`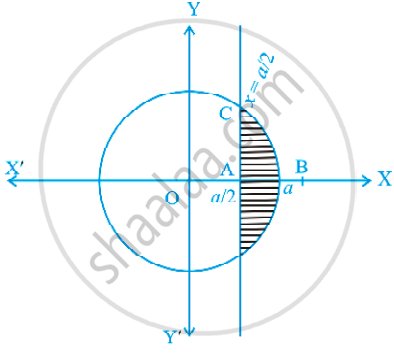
Calcualte the area under the curve y = `2sqrt(x)` included between the lines x = 0 and x = 1
Draw a rough sketch of the curve y = `sqrt(x - 1)` in the interval [1, 5]. Find the area under the curve and between the lines x = 1 and x = 5.
Determine the area under the curve y = `sqrt("a"^2 - x^2)` included between the lines x = 0 and x = a.
Area lying between the curves `y^2 = 4x` and `y = 2x`
Let the area enclosed by the x-axis, and the tangent and normal drawn to the curve 4x3 – 3xy2 + 6x2 – 5xy – 8y2 + 9x + 14 = 0 at the point (–2, 3) be A. Then 8A is equal to ______.
Using Integration, find the area of triangle whose vertices are (– 1, 1), (0, 5) and (3, 2).
Find the area enclosed between 3y = x2, X-axis and x = 2 to x = 3.
Find the area cut off from the parabola 4y = 3x2 by the line 2y = 3x + 12.
