Advertisements
Advertisements
प्रश्न
The following figure shows a square cardboard ABCD of side 28 cm. Four identical circles of the largest possible sizes are cut from this card as shown below.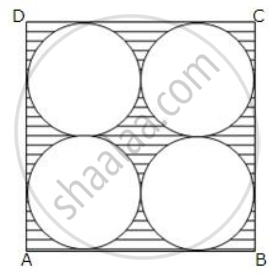
Find the area of the remaining card-board.
उत्तर
Area of the square is given by
A = 282
= 784 cm2
Since there are four identical circles inside the square.
Hence the radius of each circle is one-fourth of the side of the square.
Area of one circle = π x 72
= 154 cm2
Area of four circle = 4 x 154cm2
= 616 cm2
Area remaining = 784 - 616
= 168 cm2
The area remaining in the cardboard is = 168 cm2.
APPEARS IN
संबंधित प्रश्न
One side of a rectangle is 12 cm long and its diagonal measure 37 cm. Find the other side and the area of the rectangle.
A room is 16 m long and 13.5 m broad. Find the cost of covering its floor with 75-m-wide carpet at ₹ 60 per metre.
The area of rectangle is `192cm^2` and its perimeter is 56 cm. Find the dimensions of the rectangle.
A carpet is laid on floor of a room 8 m by 5 m. There is border of constant width all around the carpet. If the area of the border is `12m^2` find its width.
Two circular pieces of equal radii and maximum area, touching each other are cut out from a rectangular card board of dimensions 14 cm × 7 cm. Find the area of the remaining card board. (Use π = 22/7).
The area of the largest triangle that can be inscribed in a semi-circle of radius r is
If area of a circle inscribed in an equilateral triangle is 48π square units, then perimeter of the triangle is
A wire is bent to form a square enclosing an area of 484 cm2. Using the same wire, a circle is formed. Find the area of the circle.
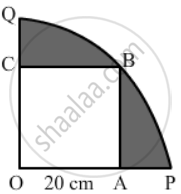
In the given figure, a square OABC has been inscribed in the quadrant OPBQ. If OA = 20 cm, then the area of the shaded region is
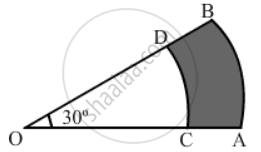
In the given figure, the sectors of two concentric circles of radii 7 cm and 3.5 cm are shown. Find the area of the shaded region.