Advertisements
Advertisements
प्रश्न
The frustum shaped outer portion of the table lamp has to be painted including the top part. Find the total cost of painting the lamp if the cost of painting 1 sq.cm is ₹ 2.
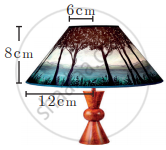
उत्तर
Slant height of the frustum (l)
= `sqrt("h"^2 + ("R" - "r")^2`
= `sqrt(8^2 + (12 - 6)^2`
= `sqrt(64 + (6)^2`
= `sqrt(64 + 36)`
= `sqrt(100)`
Slant height = 10 m
Total Area to be painted = C.S.A of the Frustum + top area
= πl (R + r) + πr2 sq.units
= π[l (R + r) + r2]
= `22/7[10(12 + 6) + 6^2]`
= `22/7[10 xx 18 + 36] "cm"^2`
= `22/7[180 + 36] "cm"^2`
= `(22 xx 216)/7`
= `4752/7 "cm"^2`
= 678.86 cm2
Cost of painting = ₹ 678.86 × 2 = ₹ 1357.72
APPEARS IN
संबंधित प्रश्न
A solid consists of a circular cylinder with an exact fitting right circular cone placed at the top. The height of the cone is h. If the total volume of the solid is 3 times the volume of the cone, then the height of the circular is
The diameters of the ends of a frustum of a cone are 32 cm and 20 cm. If its slant height is 10 cm, then its lateral surface area is
In a right circular cone , the cross-section made by a plane parallel to the base is a
A container in the shape of a frustum of a cone having diameters of its two circular faces as 35 cm and 30 cm and vertical height 14 cm,
is completely filled with oil. If each cm3 of oil has mass 1.2 g, then find the cost of oil in the container if it costs ₹40 per kg.
A bucket is in the form of a frustum of a cone with a capacity of 12308.8 cm3 of water. The radii of the top and bottom circular ends are
20 cm and 12 cm, respectively. Find the height of the bucket. [Use π = 3.14]
A metallic spherical shell of internal and external diameters 4 cm and 8 cm, respectively, is melted and recast in the form of a cone of base diameter 8 cm. The height of the cone is ______.
A shuttlecock used for playing badminton has the shape of the combination of ______.
The number of conical bottles of radius 2 cm and height 3.6 cm, required to empty the liquid from a cylindrical bottle of radius 6 cm and height 10 cm is ______.
A metallic spherical shell of internal and external diameters 4 cm and 8 cm, respectively is melted and recast into the form a cone of base diameter 8 cm. The height of the cone is ______.
The diameters of the two circular ends of the bucket are 44 cm and 24 cm. The height of the bucket is 35 cm. The capacity of the bucket is ______.
