Advertisements
Advertisements
प्रश्न
The horizontal range of a projectile fired at an angle of 15° is 50 m. If it is fired with the same speed at an angle of 45°, its range will be ______.
पर्याय
60 m
71 m
100 m
141 m
उत्तर
The horizontal range of a projectile fired at an angle of 15° is 50 m. If it is fired with the same speed at an angle of 45°, its range will be 100 m.
Explanation:
We know that
Where θ is angle of projection
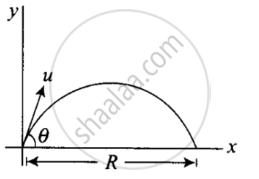
Given, θ = 15° and R = 50 m
Range, R = `(u^2 sin 2 θ)/g`
Putting all the given values in the formula, we get
⇒ R = `50 m = (u^2 sin(2 xx 15^circ))/g`
⇒ `50 xx g = u^2 sin 30^circ = u^2 xx 1/2`
⇒ `50 xx g xx 2 = u^2`
⇒ `u^2 = 50 xx 9.8 xx 2 = 100 xx 9.8` = 980
⇒ `u = sqrt(980)`
= `sqrt(49 xx 20)`
= `7 xx 2 xx sqrt(5)` m/s
= `14 xx 2.23` m/s
= 31.304 m/s
For θ = 45°, R = `(u^2 sin 2 xx 45^circ)/g = u^2/g` ......(∵ sin 90° = 1)
⇒ R = `(14sqrt(5))^2/g`
= `(14 xx 14 xx 5)/9.8`
= 100 m
APPEARS IN
संबंधित प्रश्न
A bullet fired at an angle of 30° with the horizontal hits the ground 3.0 km away. By adjusting its angle of projection, can one hope to hit a target 5.0 km away? Assume the muzzle speed to the fixed, and neglect air resistance.
A cricket ball thrown across a field is at heights h1 and h2 from the point of projection at times t1 and t2 respectively after the throw. The ball is caught by a fielder at the same height as that of projection. The time of flight of the ball in this journey is
A ball rolls of the top of the stairway with a horizontal velocity u ms−1. If the steps are h m high and b m wide, the ball will hit the edge of the nth step, if:
A particle moving in a circle of radius R with a uniform speed takes a time T to complete one revolution.
If this particle were projected with the same speed at an angle ‘θ’ to the horizontal, the maximum height attained by it equals 4R. The angle of projection, θ, is then given by ______.
In dealing with motion of projectile in air, we ignore effect of air resistance on motion. This gives trajectory as a parabola as you have studied. What would the trajectory look like if air resistance is included? Sketch such a trajectory and explain why you have drawn it that way.
If a baseball player can throw a ball at maximum distance = d over a ground, the maximum vertical height to which he can throw it, will be (Ball has same initial speed in each case):
A body starts from rest with constant acceleration a, its velocity after n second is v. The displacement of body in last two seconds is ______.
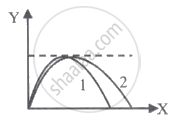
The trajectories of the two projectiles are shown in the figure. Let T1 and T2 be the time periods and u1 and u2 be their speeds of projection. Then ______.
An object is projected in the air with initial velocity u at an angle θ. The projectile motion is such that the horizontal range R, is maximum.
Another object is projected in the air with a horizontal range half of the range of first object. The initial velocity remains same in both the case. The value of the angle of projection, at which the second object is projected, will be ______ degree.
A person throws a ball with a speed of 10 m/s at an angle of 30° with horizontally from the top of a 10 m high tower. The distance of the ball from the foot of the tower after falling on the ground will be ______.
