Advertisements
Advertisements
प्रश्न
The line joining P (-5, 6) and Q (3, 2) intersects the y-axis at R. PM and QN are perpendiculars from P and Q on the x-axis. Find the ratio PR: RQ.
उत्तर
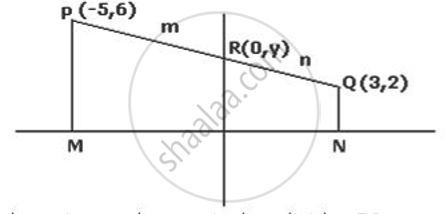
R(O, y) is the point on the y-axis that divides PQ.
Let the ratio in which PQ is divided by R be m:n.
Now, R(o,y),(x1,y1)=(-5,6) and (x2,y2)=(3,2) and the ratio is m:n.
`0 = ("m x"_2 + "nx"_1)/("m + n")`
`=> 0 = (3"m" - 5"n")/("m + n")`
⇒ 0 = 3m = 5n
⇒ 3m = 5n
`=> "m"/"n" = 5/3`
⇒ m : n = 5 : 3
⇒ PR : RQ = 5 : 3
APPEARS IN
संबंधित प्रश्न
If the point C (–1, 2) divides internally the line segment joining A (2, 5) and B in ratio 3 : 4, find the coordinates of B
Find the coordinates of the centroid of a triangle whose vertices are (–1, 0), (5, –2) and (8, 2)
Find the coordinates of the points of trisection of the line segment joining (4, -1) and (-2, -3).
Calculate the ratio in which the line joining the points (–3, –1) and (5, 7) is divided by the line x = 2. Also, find the co-ordinates of the point of intersection.
The line joining P(–4, 5) and Q(3, 2) intersects the y-axis at point R. PM and QN are perpendicular from P and Q on the x-axis Find:
- the ratio PR : RQ
- the coordinates of R.
- the area of the quadrilateral PMNQ.
M and N are two points on the X axis and Y axis respectively. P (3, 2) divides the line segment MN in the ratio 2 : 3.
Find:
(i) the coordinates of M and N
(ii) slope of the line MN.
In what ratio is the line joining (2, -4) and (-3, 6) divided by the line y = O ?
The perpendicular bisector of the line segment joining the points A(1, 5) and B(4, 6) cuts the y-axis at ______.
If the points A(1, 2), O(0, 0), C(a, b) are collinear, then ______.
If P(9a – 2, – b) divides line segment joining A(3a + 1, –3) and B(8a, 5) in the ratio 3 : 1, find the values of a and b.
