Advertisements
Advertisements
प्रश्न
There are two temples, one on each bank of a river, just opposite to each other. One temple is 50 m high. From the top of this temple, the angles of depression of the top and the foot of the other temple are 30° and 60° respectively. Find the width of the river and the height of the other temple.
उत्तर
Let AB and CE are two temples each at the bank of the river. The top of the temple CE makes an angle of depressions at the top and bottom of tower AB are 30° and 60°
Let CE = 50 m and AB = H m and ∠CBE = 60°, ∠DAE = 30°
The corresponding figure is as follows
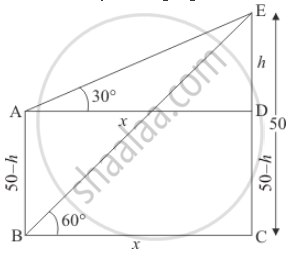
In Δ ADE
`=> tan 30^@ = h/x`
`=> 1/sqrt3 = h/x`
`=> x = hsqrt3`
Again in ΔBCE
`=> tan 60^@ = 50/x`
`=> sqrt3 = 50/x`
`=> 50 = sqrt3 xxx hsqrt3`
`=> h = 50/3`
Now the distance between the temples
`x = hsqrt3`
`= 50/3xx sqrt3`
`= 50/3`
Therefore `H = 50 - 50/3`
`=> H = 33.33
Hence distance between the temples is `50/sqrt3 m = 28.83 m` and height of temple is 33.33 m
APPEARS IN
संबंधित प्रश्न
A bus travels at a certain average speed for a distance of 75 km and then travels a distance of 90 km at an average speed of 10 km/h more than the first speed. If it takes 3 hours to complete the total journey, find its first speed?
The length of the shadow of a tower standing on the level plane is found to 2x meter longer when the sun's altitude is 30° than when it was 45°. Prove that the height of the tower is `x(sqrt3 + 1)` meters.
The angle of elevation on the top of a building from the foot of a tower is 30° . The angle of elevation of the top of the tower when seen from the top of the second water is 60° .If the tower is 60m high, find the height of the building.
An electrician has to repair an electric fault on a pole of height 4 meters. He needs to reach a point 1 meter below the top of the pole to undertake the repair work. What should be the length of the ladder that he should use, which when inclined at an angle of 60° to the horizontal would enable him to reach the required position?
A tower subtends an angle of 30° at a point on the same level as its foot. At a second point h metres above the first, the depression of the foot of the tower is 60°. The height of the tower is
From the top of a lighthouse, an observer looks at a ship and finds the angle of depression to be 60° . If the height of the lighthouse is 84 meters, then find how far is that ship from the lighthouse? (√3 = 1.73)
A man in a boat rowing away from a lighthouse 100 m high takes 2 minutes to change the angle of elevation of the top of the lighthouse from 60° to 30°.
Find the speed of the boat in metres per minute. [Use `sqrt(3` = 1.732]Use 3=1.732">
The angles of elevation of the top of the rock from the top and foot of 100 m high tower are respectively 30° and 45°. The height of the rock is ____________.
A tower stands vertically on the ground. From a point on the ground, which is 30 m away from the foot of the tower, the angle of elevation of the top of the tower is found to be 30°. Find the height of the tower.
The angle of elevation of the top of a tower 30 m high from the foot of another tower in the same plane is 60°, then the distance between the two towers is ____________.
