Advertisements
Advertisements
प्रश्न
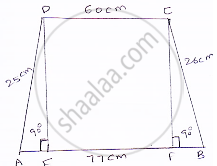
Two parallel side of a trapezium are 60 cm and 77 cm and other sides are 25 cm and 26 cm. Find the area of the trapezium.
उत्तर
Given that two parallel sides of trapezium are AB = 77 and CD = 60 cm
Other sides are BC = 26 m and AD = 25 cm.
Join AE and CF
Now, DE ⊥ AB and CF ⊥ AB
∴ DC = EF = 60 cm
Let AE = x
⇒ BF = 77 – 60 – x = 17 – x
`In ΔADE, DE^2 = AD^2 – AE^2 = 25^2 – x^2` [∵ Pythagoras theorem]
And in ΔBCF, `CF^2= BC^2 – BF^2` [∵ By Pythagoras theorem]
`⇒25=sqrt(26^2-(17-x)^2)`
`⇒25^2-x^2=25^2-(289-x^2-34-x)` [ ∵`(a-b)^2=a^2-2ab+b^2` ]
`⇒265-x^2=676-289-x^2+34x`
`34x=238`
`x=7`
`∴ DE =sqrt(25^2-x^2)=sqrt(625-7^2)=sqrt(516)=24cm`
∴ Area of trapezium = `1/2`(𝑠𝑢𝑚 𝑜𝑓 𝑝𝑎𝑟𝑎𝑙𝑙𝑒𝑙 𝑠𝑖𝑑𝑒𝑠)×ℎ𝑒𝑖𝑔ℎ𝑡=`1/2`(60×77)×24=`1644cm^2`
APPEARS IN
संबंधित प्रश्न
A field is in the shape of a trapezium whose parallel sides are 25 m and 10 m. The non-parallel sides are 14 m and 13 m. Find the area of the field.
Find the area of a rhombus whose perimeter is 80 m and one of whose diagonal is 24 m.
A square and an equilateral triangle have equal perimeters. If the diagonal of the square is \[12\sqrt{2}\] cm, then area of the triangle is
A park is in the shape of a quadrilateral. The sides of the park are 15 m, 20 m, 26 m and 17 m and the angle between the first two sides is a right angle. Find the area of the park
The adjacent sides of a parallelogram measures 34 m, 20 m and the measure of the diagonal is 42 m. Find the area of parallelogram
The area of an equilateral triangle with side `2sqrt(3)` cm is ______.
The length of each side of an equilateral triangle having an area of `9sqrt(3)`cm2 is ______.
If the area of an equilateral triangle is `16sqrt(3)` cm2, then the perimeter of the triangle is ______.
In a triangle, the sides are given as 11 cm, 12 cm and 13 cm. The length of the altitude is 10.25 cm corresponding to the side having length 12 cm.
