Advertisements
Advertisements
प्रश्न
Two points A and B are on the same side of a tower and in the same straight line with its base. The angles of depression of these points from the top of the tower are 60° and 45° respectively. If the height of the tower is 15 m, then find the distance between the points.
उत्तर
Let CD be the tower. A and B are the two points on the same side of the tower.
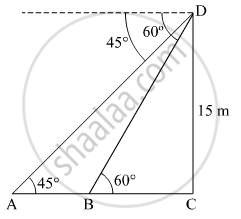
In ΔDBC
`tan 60^@ = (DC)/(BC)`
`=> sqrt3 = 15/(BC)`
`=> BC = 15/sqrt3`
`=> BC = 5sqrt3 m`
In ΔDAC
`tan 45^@ = (DC)/(AC)`
`=> 1 = 15/"AC"`
`=> AC = 15 m`
Now
AC = AB + BC
`:. AB = AC - BC = 15 - 5sqrt3 = 5(3 - sqrt3) m`
Hence, the distance between the two points A and B is `5(3 - sqrt3) m`
APPEARS IN
संबंधित प्रश्न
A circus artist is climbing a 20 m long rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole, if the angle made by the rope with the ground level is 30°.

The ratio of the height of a tower and the length of its shadow on the ground is `sqrt3 : 1`. What is the angle of elevation of the sun?
A 1.6 m tall girl stands at a distance of 3.2 m from a lamp-post and casts a shadow of 4.8 m on the ground. Find the height of the lamp-post by using (i) trigonometric ratios (ii) property of similar triangles.
The angle of elevation of the top of a chimney form the foot of a tower is 60° and the angle of depression of the foot of the chimney from the top of the tower is 30° . If the height of the tower is 40 meters. Find the height of the chimney.
A kite is flying at a height of 45 m above the ground. The string attached to the kite is temporarily tied to a point on the ground. The inclination of the string with the ground is
60°. Find the length of the string assuming that there is no slack in the string.
If two circles having centers P and Q and radii 3 cm and 5 cm. touch each other externally, find the distance PQ.
If two towers of heights h1 and h2 subtend angles of 60° and 30° respectively at the mid-point of the line joining their feet, then h1: h2 = ____________.
If a man standing on a platform 3 metres above the surface of a lake observes a cloud and its reflection in the lake, then the angle of elevation of the cloud is equal to the angle of depression of its reflection.
Find the angle of elevation of the sun when the shadow of a pole h metres high is `sqrt(3)` h metres long.
From the top of building AB, a point C is observed on the ground whose angle of depression is 60° and which is at a distance of 40 m from the base of the building. Complete the following activity to find the height of building AB.

From figure, BC = `square`, ∠ACB = `square`
In ΔACB,
tan `square = square/(BC)`
⇒ `square = square/square`
⇒ `square = square`
Hence, the height of the building AB is `square`.
