Question

Two trees 7 m and 4 m high stand upright on a ground. If their bases (roots) are 4 m apart, then the distance between their tops is ______.

पर्याय

• 3 m

• 5 m

• 4 m

• 11 m

Answer 1

Two trees 7 m and 4 m high stand upright on a ground. If their bases (roots) are 4 m apart, then the distance between their tops is 5 m.

Explanation:

Let BE be the smaller tree and AD be the bigger tree. Now, we have to find AB (i.e. the distance between their tops).

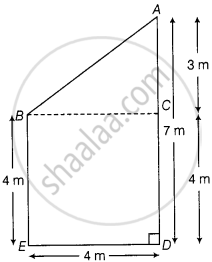
By observing,

ED = BC = 4 m and BE = CD = 4 m

In ΔABC, BC = 4 m

And AC = AD – CD = (7 – 4) m = 3 m

In right angled ΔABC,

AB² = AC² + BC² = 4² + 3²  ......[By Pythagoras theorem]

= 16 + 9

⇒ AB² = 25

⇒ AB = `sqrt(25)`

⇒ AB = 5 m

Therefore, the distance between their tops is 5 m.