Advertisements
Advertisements
प्रश्न
Use ruler and compasses only for the following questions:
Construct triangle BCP, when CB = 5 cm, BP = 4 cm, ∠PBC = 45°.
Complete the rectangle ABCD such that :
(i) P is equidistant from AB and BC and
(ii) P is equidistant from C and D. Measure and write down the length of AB.
उत्तर
Given: BC = 5 cm, BP = 4 cm and ∠PBC = 45°
Steps of construction :
1. Constant ΔBCP with BC = 5 cm, BP = 4 cm and ∠PBC = 45°.
2. Draw perpendicular BE and CF and B and C respectively.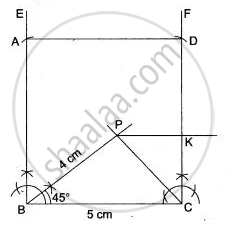
3. Draw perpendicular from on CF meeting CF in K.
4. Cut CD from CF, such that CK = KD.
5. Cut BA from BE, such that BA = CD.
6. Join AD.
Hence, ABCD is the required rectangle and AB = 5·7 cm.
APPEARS IN
संबंधित प्रश्न
Construct a triangle ABC with AB = 5.5 cm, AC = 6 cm and ∠BAC = 105°
Hence:
1) Construct the locus of points equidistant from BA and BC
2) Construct the locus of points equidistant from B and C.
3) Mark the point which satisfies the above two loci as P. Measure and write the length of PC.
O is a fixed point. Point P moves along a fixed line AB. Q is a point on OP produced such that OP = PQ. Prove that the locus of point Q is a line parallel to AB.
Construct a ti.PQR, in which PQ=S. 5 cm, QR=3. 2 cm and PR=4.8 cm. Draw the locus of a point which moves so that it is always 2.5 cm from Q.
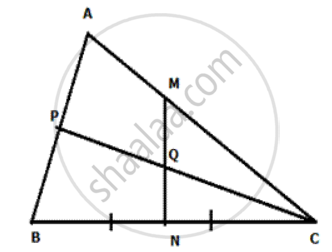
In the given figure ABC is a triangle. CP bisects angle ACB and MN is perpendicular bisector of BC. MN cuts CP at Q. Prove Q is equidistant from B and C, and also that Q is equidistant from BC and AC.
Draw and describe the lorus in the following cases:
The lorus of points inside a circle and equidistant from two fixed points on the circle .
Draw and describe the lorus in the following cases:
The lorus of a point in rhombus ABCD which is equidistant from AB and AD .
Describe completely the locus of point in the following cases:
Midpoint of radii of a circle.
Describe completely the locus of points in the following cases:
Point in a plane equidistant from a given line.
State and draw the locus of a swimmer maintaining the same distance from a lighthouse.
Construct a Δ ABC, with AB = 6 cm, AC = BC = 9 cm; find a point 4 cm from A and equidistant from B and C.
