SSC (English Medium)
SSC (Marathi Semi-English)
Academic Year: 2018-2019
Date: मार्च 2019
Advertisements
The co-ordinates of point A and B are 4 and -8 respectively. Find d(A, B).
Chapter: [0.05] Co-ordinate Geometry
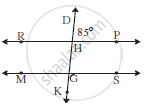
In the adjoining figure line RP ||line MS , line DK is a transversal . If ∠DHP = 85° find ∠RHG and ∠HGS.
Chapter: [0.05] Co-ordinate Geometry
∠ACD is an exterior angle of Δ ABC. If ∠B = 40o, ∠A = 70o find ∠ACD.

Chapter: [0.06] Trigonometry
Digonals of parallelogram WXYZ intersect at point O. If OY =5, find WY.
Chapter: [0.02] Pythagoras Theorem
In which qudrant does point A(-3, 2) lie?
On which axis does point B(12, 0) lie?
Chapter: [0.03] Circle
Find the curved surface area of a sphere of radius 1cm. (π = 3.14)
Chapter: [0.07] Mensuration
Simplify : 2 sin30 + 3 tan45.
Chapter: [0.06] Trigonometry
In the adjoining figure, point O is the centre of the cirlcle, seg OM ⊥ chord AB. If OM = 8cm, AB = 12 cm, then find OB.

Chapter: [0.03] Circle
In ΔPQR, PQ = 10 cm, QR = 12cm, PR = 8 cm, find the biggest and the smallest angle of the triangle.
Chapter: [0.01] Similarity
Advertisements
How many common tangents can be drawn to two circles which touch each other internally?
(A) One (B) Two (C) Three (D) Four
Chapter: [0.03] Circle
Distance of point (-3, 4) from the origin is .....
(A) 7 (B) 1 (C) 5 (D) 4
Chapter: [0.05] Co-ordinate Geometry
Four alternative answers for the following question are given. Choose the correct alternative and write its alphabet:
sin θ × cosec θ = ______
1
0
`1/2`
`sqrt2`
Chapter: [0.06] Trigonometry
Measure of an arc of a sector of a circle is 900 and its radius is 7cm. Find the perimeter of the sector.
(A) 44 cm (B) 25 cm (C) 36 cm (D) 56 cm
Chapter: [0.07] Mensuration
ΔABC ∼ ΔDEF and A(ΔABC) : A Δ(DEF) = 1 : 2 If AB = 4 find DE.
Chapter: [0.01] Similarity
In the given figure, m(arc NS) = 125°, m(arc EF) = 37°, find the measure ∠NMS.
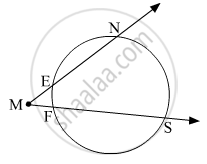
Chapter: [0.03] Circle
Find th co-ordinates of the midpoint of the line segment joining P(0, 6) and Q(12, 20).
Chapter: [0.05] Co-ordinate Geometry
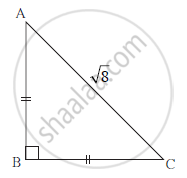
With the help of the information given in the figure, fill in the boxes to find AB and BC .
AB = BC (Given)
∴∠ BAC = ∠ BCA =
∴ AB = BC = × AC
= × `sqrt8`
= × `2sqrt2`
= 2
Chapter: [0.01] Similarity
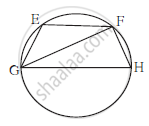
In the adjoining figure chord EF || chord GH.
Prove that chord EG ≅ chord FH.
Fill in the boxes and write the complete proof.
Chapter: [0.03] Circle
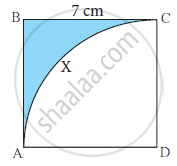
Side of square ABCD is 7 cm. With D as the centre and DA as radius, arc AXC is drawn.Find the area of the shaded region with the help of the following flow chart .

Chapter: [0.03] Circle
Advertisements

In ΔMNP, ∠MNP = 90˚, seg NQ ⊥ seg MP, MQ = 9, QP = 4, find NQ.
Chapter: [0.02] Pythagoras Theorem
Prove that secθ + tanθ =`(costheta)/(1-sintheta)`.
Chapter: [0.06] Trigonometry

Radii of the top and the base of a frustum of a cone are 5 cm and 2 cm respectively. Its height is 9 cm. Find its volume. (π = 3.14)
Chapter: [0.06] Trigonometry
Prove that :
“If a line parallel to a side of a triangle intersects the remaining sides in two distince points, then the line divides the sides in the same proportion.”
Chapter: [0.05] Co-ordinate Geometry
Draw a circle with centre O and radius 3.5 cm. Take point P at a distance 5.7 cm from the centre. Draw tangents to the circle from point P.
Chapter: [0.04] Geometric Constructions
Line PQ is parallel to line RS where points P,Q,R and S have
co-ordinates (2, 4), (3, 6), (3, 1) and (5, k) respectively. Find value of k.
Chapter: [0.05] Co-ordinate Geometry
From the top of a light house, an abserver looking at a boat makes an angle of depression of 600. If the height of the lighthouse is 90 m then find how far is the boat from the lighthouse. (3 = 1.73)
Chapter: [0.01] Similarity
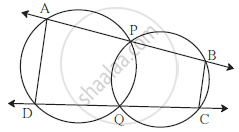
Two circles intersect each other at points P and Q. Secants drawn through P and Q intersect the circles at points A,B and D,C. Prove that : ∠ADC + ∠BCD = 180°
Chapter: [0.03] Circle
ΔXYZ ∼ ΔPYR; In ΔXYZ, ∠Y = 60o, XY = 4.5 cm, YZ = 5.1 cm and XYPY =` 4/7` Construct ΔXYZ and ΔPYR.
Chapter: [0.05] Co-ordinate Geometry

O is any point in the interior of ΔABC. Bisectors of ∠AOB, ∠BOC and ∠AOC intersect side AB, side BC, side AC in
F, D and E respectively.
Prove that
BF × AE × CD = AF × CE × BD
Chapter: [0.01] Similarity
There is a hemispherical bowl. A cone is to be made such that, if it is filled with water twice and the water is poured in the bowl, it will be filled just completely. State how will you decide the radius and perpendicular height of the cone.
Chapter: [0.03] Circle
Other Solutions
Submit Question Paper
Help us maintain new question papers on Shaalaa.com, so we can continue to help studentsonly jpg, png and pdf files
Maharashtra State Board previous year question papers 10th Standard Board Exam Geometry Mathematics 2 with solutions 2018 - 2019
Previous year Question paper for Maharashtra State Board 10th Standard Board Exam Geometry Maths 2-2019 is solved by experts. Solved question papers gives you the chance to check yourself after your mock test.
By referring the question paper Solutions for Geometry Mathematics 2, you can scale your preparation level and work on your weak areas. It will also help the candidates in developing the time-management skills. Practice makes perfect, and there is no better way to practice than to attempt previous year question paper solutions of Maharashtra State Board 10th Standard Board Exam.
How Maharashtra State Board 10th Standard Board Exam Question Paper solutions Help Students ?
• Question paper solutions for Geometry Mathematics 2 will helps students to prepare for exam.
• Question paper with answer will boost students confidence in exam time and also give you an idea About the important questions and topics to be prepared for the board exam.
• For finding solution of question papers no need to refer so multiple sources like textbook or guides.
