Advertisements
Advertisements
Question
A bulb is made using two filaments. A switch selects whether the filaments are used individually or in parallel. When used with a 15 V battery, the bulb can be operated at 5 W, 10 W or 15 W. What should be the resistances of the filaments?
Solution
We know:-
\[R = \frac{V^2}{P}\]
where R is the resistance, V is the voltage drop and P is the power on which the bulb is operated.
We can calculate the resistance of the bulb for the same V and different P.
The resistances of the bulb for three different powers are:-
\[R_1 = \frac{\left( 15 \right)^2}{5} = 45 \Omega\]
\[ R_2 = \frac{\left( 15 \right)^2}{10} = 22 . 5 \Omega\]
\[ R_3 = \frac{\left( 15 \right)^2}{15} = 15 \Omega\]
When the two resistance are used in parallel, the equivalent resistance will be less than their individual resistance.
∴ The two resistance are 45 Ω and 22.5 Ω.
APPEARS IN
RELATED QUESTIONS
Two electric bulbs P and Q have their resistances in the ratio of 1 : 2. They are connected in series across a battery. Find the ratio of the power dissipation in these bulbs
A uniform wire of resistance 50 Ω is cut into 5 equal parts. These parts are now connected in parallel. The equivalent resistance of the combination is ______________ .
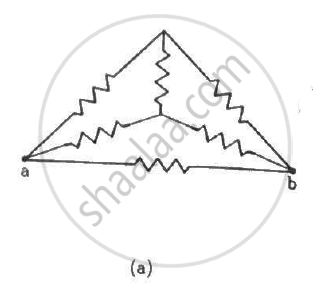
Find the equivalent resistance of the circuits shown in the figure between the points a and b. Each resistor has resistance r.

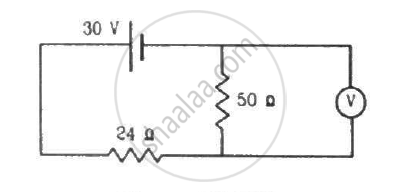
The voltmeter shown in the figure reads 18 V across the 50 Ω resistor. Find the resistance of the voltmeter.
Two voltameters, one with a solution of silver salt and the other with a trivalent-metal salt, are connected in series and a current of 2 A is maintained for 1.50 hours. It is found that 1.00 g of the trivalent metal is deposited. (a) What is the atomic weight of the trivalent metal?
(b) How much silver is deposited during this period? Atomic weight of silver is 107.9 g mol−1.
Two resistors R1= 60 Ω and R2 = 90Ω are connected in parallel. If electric power consumed by the resistor R1 is15 W, calculate the power consumed by the resistor R2.
A solenoid L and a resistor R are connected in series to a battery, through a switch. When the switch is put on, current I flowing through it varies with time t as shown in which of the graphs given below:
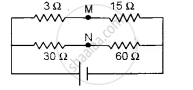
How much resistance should be connected to 15 Ω resistor shown in the circuit in figure below so that the points M and N are at the same potential:
An ammeter together with an unknown resistance in series is connected across two identical batteries each of emf 1.5 V. When the batteries are connected in series, the galvanometer records a current of 1A and when the batteries are in parallel, the current is 0.6A. Then the internal resistance of the battery is ______.
Assertion (A): To increase the range of an ammeter, we must connect a suitable high resistance in series to it.
Reason (R): The ammeter with increased range should have high resistance.
Select the most appropriate answer from the options given below:
If two resistors of resistances R1 = (4 ± 0.5) Ω and R2 = (16 ± 0.5) Ω are connected in series. The eqivalent resistance with the limits of percentage error is ______.
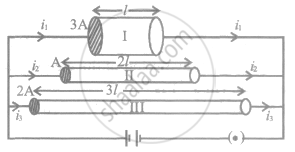
The figure shows three conductors I, II and III of same material, different lengths l, 2l and 3l and of different areas of cross-sections 3A, A and 2A respectively. Arrange them in the increasing order of current drawn from the battery.
The effective resistance of a parallel connection that consists of four wires of equal length, equal area of cross-section and the same material is 0.25 Ω. What will be the effective resistance if they are connected in series?
Let there be n resistors R1............Rn with Rmax = max (R1......... Rn) and Rmin = min {R1..... Rn}. Show that when they are connected in parallel, the resultant resistance RP < R min and when they are connected in series, the resultant resistance RS > Rmax. Interpret the result physically.
- Assertion (A): The equivalent resistance between points A and B in the given networks is 2R.
- Reason (R): All the resistors are connected in parallel.
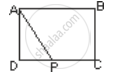
A wire of uniform cross-section and resistance 4 ohms is bent in the shape of square ABCD. Point A is connected to point P on DC by a wire AP of resistance 1 ohm. When a potential difference is applied between A and C, the points B and P are seen to be at the same potential. What is the resistance of the part DP?
Eight identical cells, each of emf 2V and internal resistance 3 Ω, are connected in series to form a row. Six such rows are connected in parallel to form a battery. This battery is now connected to an external resistor R of resistance 6 Ω. Calculate:
- emf of the battery.
- internal resistance of the battery.
- current flowing through R.
