Advertisements
Advertisements
Question
A canvas tent is in the shape of a cylinder surmounted by a conical roof. The common diameter of the cone and the cylinder is 14 m. The height of the cylindrical part is 8 m and the height of the conical roof is 4 m. Find the area of the canvas used to make the tent.
Solution
Height of the cylindrical part = H = 8 m
Height of the conical part = h = 4 m
Diameter = 14 m ⇒ radius = r = 7 m
Slant height of the cone = I =
l = `sqrt(r^2 + h^2)`
l = `sqrt(7^2 + 4^2)`
l = `sqrt(65) = 8.06` m
Slant height of cone = 8.06 m
Area of the canvas used = Curved surface area of cylinder + curved surface area of cone
= `2pirH + pirl`
= `(2 xx 22/7 xx 7 xx 8) + (22/7 xx 7 xx 8.06)`
= 352 + 177.32
= 529.32 m2
Area of the canvas used = 529.32 m2
APPEARS IN
RELATED QUESTIONS
An elastic belt is placed around the rim of a pulley of radius 5 cm. (Fig. 10) From one point C on the belt, the elastic belt is pulled directly away from the centre O of the pulley until it is at P, 10 cm from the point O. Find the length of the belt that is still in contact with the pulley. Also find the shaded area (use π = 3.14 and `sqrt3=1.73)`

The minute hand of a clock is 10cm long. Find the area of the face of the clock described by the minute hand between 9 A.M. and 9.35 A.M.
Find the circumference of the circle with the following radius: (Take `bb(pi = 22/7`)
21 cm
The outer circumference of a circular race track is 528m. The track is everywhere 14m
wide. Calculate the cost of leveling the track at rate of 50 paise per square metre.
The shape of the cross section of a canal is a trapezium. If the canal is 10 m wide at the top, 6 m wide at the bottom and the area of its cross section is `640m^2` , find the depth of the canal.
The circumference of a circle is 22 cm. Find the area of its quadrant.
The areas of two circles are in the ratio 4: 9. What is the ratio between their circumferences?
In the given figure, ABCD is a square of side 4 cm. A quadrant of a circle of radius 1 cm is drawn at each vertex of the square and a circle of diameter 2 cm is also drawn. Find the area of the shaded region. [π = 3.14]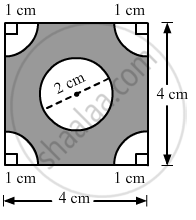
In a circle of radius 10.5 cm, the minor arc is one-fifth of the major arc. Find the area of the sector corresponding to the major arc.
The area of a circle inscribed in an equilateral triangle is 154 cm2. Find the perimeter of the triangle.
The circumference of a circle is equal to the perimeter of a square. The area of the square is 484 sq. m. Find the area of the circle.
The radius of a circle is 5 m. Find the circumference of the circle whose area is 49 times the area of the given circle.
Construct an angle PQR = 45°. Mark a point S on QR such that QS = 4.5 cm. Construct a circle to touch PQ at Q and also to pass through S.
If the circumference of a circle is 352 metres, then its area in square metres is ____________.
Areas of two circles are equal. Is it necessary that their circumferences are equal? Why?
The area of a circular playground is 22176 m2. Find the cost of fencing this ground at the rate of Rs 50 per metre.
In reference to a circle the value of π is equal to ______.
The area of a square is 100 cm2. The circumference (in cm) of the largest circle cut of it is ______.
Circumference ‘C’ of a circle can be found by multiplying diameter ‘d’ with ______.
