Advertisements
Advertisements
Question
In the given figure, ABCD is a square of side 4 cm. A quadrant of a circle of radius 1 cm is drawn at each vertex of the square and a circle of diameter 2 cm is also drawn. Find the area of the shaded region. [π = 3.14]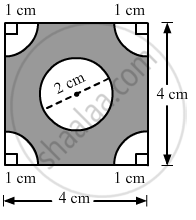
Solution
Area of the square ABCD =(Side)2
= 42
= 16 cm2
Area of the circle = πr2
Radius = 1 cm
Area = 3.14 × (1)2
= 3.14 cm2
Area of the quadrant of one circle`=1/4pi"r"^2`
`=1/4xx3.14xx1^2`
= 0.785 cm2
Area of the quadrants of four circles = 0.785 × 4 = 3.14 cm2
Area of the shaded region = Area of the square - Area of the circle - Area of the quadrants of four circles = 16-3.14-3.14 = 9.72 cm2
APPEARS IN
RELATED QUESTIONS
Saima wants to put a lace on the edge of a circular table cover of diameter 1.5 m. Find the length of the lace required and also find its cost if one meter of the lace costs ₹ 15. (Take π = 3.14)
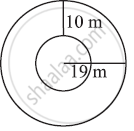
Find the circumference of the inner and the outer circles, shown in the adjoining figure? (Take π = 3.14)
The side of a square is 10 cm. find the area of circumscribed and inscribed circles.
Find the angle subtended at the centre of circle of radius 5cm by an arc of length `((5pi)/3)` cm
Find the diameter of the sphere for the following :
Volume = `523 17/21` cm3
Find the radius of the circle whose circumference is equal to the sum of the circumferences of the circles having radius 15 cm and 8 cm.
Find the diameter of a circle whose circumference is equal to the sum of circumference of circles with radius 10 cm, 12 cm, and 18 cm.
Find the area and perimeter of the circles with following: Radius = 10.5cm
In a circle of diameter 42 cm, if an arc subtends an angle of 60° at the centre, where `pi = 22/7` then length of an arc is ____________.
The numerical value of the area of a circle is greater than the numerical value of its circumference. Is this statement true? Why?
