Advertisements
Advertisements
Question
A circuit is set up by connecting inductance L = 100 mH, resistor R = 100 Ω and a capacitor of reactance 200 Ω in series. An alternating emf of \[150\sqrt{2}\] V, 500/π Hz is applies across this series combination. Calculate the power dissipated in the resistor.
Solution
Given the r.m.s value of emf in the circuit Ev = 150√2 V
The impedance of the LCR- circuit is given by
\[Z = \sqrt{R^2 + \left( X_L - X_C \right)^2}\]
\[ = \sqrt{R^2 + \left( 2\pi fL - 200 \right)^2}\]
\[ = \sqrt{{100}^2 + \left( 2\pi \times \frac{500}{\pi} \times 0 . 1 - 200 \right)^2}\]
\[ = 100\sqrt{2} \Omega\]
The r.m.s value of current Iv in the circuit is given by
\[I_v = \frac{E_v}{Z} = \frac{150\sqrt{2}}{100\sqrt{2}} = 1 . 5 A\]
Power dissipated in the resistor = EvIv = 318.2 W
APPEARS IN
RELATED QUESTIONS
Three capacitors of capacitances 2 pF, 3 pF and 4 pF are connected in parallel. Determine the charge on each capacitor if the combination is connected to a 100 V supply.
An electrical technician requires a capacitance of 2 µF in a circuit across a potential difference of 1 kV. A large number of 1 µF capacitors are available to him each of which can withstand a potential difference of not more than 400 V. Suggest a possible arrangement that requires the minimum number of capacitors.
A cylindrical capacitor has two co-axial cylinders of length 15 cm and radii 1.5 cm and 1.4 cm. The outer cylinder is earthed and the inner cylinder is given a charge of 3.5 µC. Determine the capacitance of the system and the potential of the inner cylinder. Neglect end effects (i.e., bending of field lines at the ends).
A parallel-plate capacitor has plates of unequal area. The larger plate is connected to the positive terminal of the battery and the smaller plate to its negative terminal. Let Q, and Q be the charges appearing on the positive and negative plates respectively.
A parallel-plate capacitor having plate area 20 cm2 and separation between the plates 1⋅00 mm is connected to a battery of 12⋅0 V. The plates are pulled apart to increase the separation to 2⋅0 mm. (a) Calculate the charge flown through the circuit during the process. (b) How much energy is absorbed by the battery during the process? (c) Calculate the stored energy in the electric field before and after the process. (d) Using the expression for the force between the plates, find the work done by the person pulling the plates apart. (e) Show and justify that no heat is produced during this transfer of charge as the separation is increased.
A wire of resistance 'R' is cut into 'n' equal parts. These parts are then connected in parallel with each other. The equivalent resistance of the combination is :
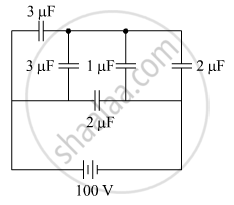
The figure shows a network of five capacitors connected to a 100 V supply. Calculate the total energy stored in the network.
An ac circuit consists of a series combination of circuit elements X and Y. The current is ahead of the voltage in phase by `pi/4`. If element X is a pure resistor of 100 Ω,
(a) name the circuit element Y.
(b) calculate the rms value of current, if rms of voltage is 141 V.
(c) what will happen if the ac source is replaced by a dc source
Three capacitors each of 4 µF are to be connected in such a way that the effective capacitance is 6µF. This can be done by connecting them:
Two charges q1 and q2 are placed at (0, 0, d) and (0, 0, – d) respectively. Find locus of points where the potential a zero.
