Advertisements
Advertisements
Question
A double-slit arrangement produces interference fringes for sodium light (λ = 589 nm) that are 0.20° apart. What is the angular fringe separation if the entire arrangement is immersed in water (n = 1.33)?
Solution
Given: θ1 = 0.20°, nw = 1.33
In the first approximation,
D sin θ1 = y1 and D sin θ2 = y2
`therefore (sin theta_2)/(sin theta_1) = "y"_2/"y"_1` ...(1)
Now, `y prop (lambdaD)/d`
For given d and D,
y ∝ λ
∴ `y_2/y_1 = lambda_2/lambda_1` ...(2)
Now, `n_w = lambda_1/lambda_2` ...(3)
From Eqs. (1), (2) and (3), we get
`(sin theta_2)/(sin theta_1) = lambda_2/lambda_1 = 1/n_w`
∴ `sin theta_2 = sin theta_1/n_w`
`sin theta_2 = (sin 0.2)/1.33`
`sin theta_2 = 0.0035/1.33`
sinθ2 = 0.0026
θ2 = sin−1 0.0026
θ2 = 9'
θ2 = 0.15°
This is the required angular fringe separation.
RELATED QUESTIONS
A narrow slit S transmitting light of wavelength λ is placed a distance d above a large plane mirror, as shown in the following figure. The light coming directly from the slit and that coming after the reflection interfere at a screen ∑ placed at a distance D from the slit. (a) What will be the intensity at a point just above the mirror, i.e. just above O? (b) At what distance from O does the first maximum occur?
The intensity at the central maximum (O) in a Young’s double slit experimental set-up shown in the figure is IO. If the distance OP equals one-third of the fringe width of the pattern, show that the intensity at point P, would equal `(I_0)/4`.

Answer the following question.
Describe any two characteristic features which distinguish between interference and diffraction phenomena. Derive the expression for the intensity at a point of the interference pattern in Young's double-slit experiment.
Answer in brief:
Explain what is the optical path length. How is it different from actual path length?
Answer in brief:
What is meant by coherent sources?
Two coherent sources whose intensity ratio is 25:1 produce interference fringes. Calculate the ratio of amplitudes of light waves coming from them.
Draw a neat labelled ray diagram of the Fresnel Biprism experiment showing the region of interference.
Obtain the relation between phase difference and path difference.
What is intensity (or) amplitude division?
Explain Young’s double-slit experimental setup and obtain the equation for path difference.
Discuss the interference in thin films and obtain the equations for constructive and destructive interference for transmitted and reflected light.
In Young’s double slit experiment, the slits are 2 mm apart and are illuminated with a mixture of two wavelength λ0 = 750 nm and λ = 900 nm. What is the minimum distance from the common central bright fringe on a screen 2 m from the slits where a bright fringe from one interference pattern coincides with a bright fringe from the other?
The interference pattern is obtained with two coherent light sources of intensity ratio n. In the interference pattern, the ratio `("I"_"max" - "I"_"min")/("I"_"max" + "I"_"min")` will be ______
A graph is plotted between the fringe-width Z and the distance D between the slit and eye-piece, keeping other adjustment same. The correct graph is
A.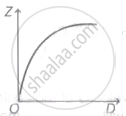 |
B. |
C.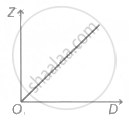 |
D.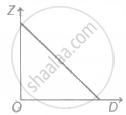 |
A metal rod has length, cross-sectional area and Young's modulus as L, A and Y, respectively. If the elongation in the rod produced is l, then work done is proportional to ______.
Band width for red light of wavelength 6600 Å is 0.42 mm. If red Light is replaced by blue light of wavelength 4400 Å, then the change m bandwidth will be ____________.
Two coherent light sources of intensity ratio 'n' are employed in an interference experiment. The ratio of the intensities of the maxima and minima in the interference pattern is (I1 > I2).
If the two slits in Young's double slit experiment have width ratio 9 : 1, the ratio of maximum to minimum intensity in the interference pattern is ______.
`phi "and" phi_2 (phi_1 > phi_2)` are the work functions of metals A and B. When light of same wavelength is incident on A and B, the fastest emitted electrons from A are ____________ those emitted from B.
In a biprism experiment, the slit separation is 1 mm. Using monochromatic light of wavelength 5000 Å, an interference pattern is obtained on the screen. Where should the screen be moved? so that the change in fringe width is 12.5 x 105 m?
In a biprism experiment, monochromatic light of wavelength (λ) is used. The distance between two coherent sources is kept constant. If the distance between slit and eyepiece (D) is varied as D1, D2, D3, and D4, the corresponding measured fringe widths are z1, z2, z3, and z4 then ______
In the biprism experiment, a source of monochromatic light is used for a certain distance between slit and eyepiece. When the distance between two virtual sources is changed from dA to dB, then the fringe width is changed from ZA to ZB. The ratio ZA to ZB is ______
Light waves from two coherent sources arrive at two points on a screen with a path difference of zero and λ/2. The ratio of the intensities at the points is ______
Young's double slit experiment is performed in water, instead of air, then fringe width ______.
Two waves with same amplitude and frequency superpose at a point. The ratio of resultant intensities when they arrive in phase to that when they arrive 90° out of phase is ______.
`[cos pi/2=0]`
In Young's double-slit experiment, the distance between the slits is 3 mm and the slits are 2 m away from the screen. Two interference patterns can be obtained on the screen due to light of wavelength 480 nm and 600 run respectively. The separation on the screen between the 5th order bright fringes on the two interference patterns is ______
In Young's double-slit experiment, the distance between the slits is 3 mm and the slits are 2 m away from the screen. Two interference patterns can be obtained on the screen due to light of wavelength 480 nm and 600 run respectively. The separation on the screen between the 5th order bright fringes on the two interference patterns is ______
Interference fringes are produced on a screen by using two light sources of intensities I and 9I. The phase difference between the beams is `pi/2` at point P and π at point Q on the screen. The difference between the resultant intensities at point P and Q is ______.
