Advertisements
Advertisements
Question
A hollow cylindrical box of length 0.5 m and area of cross-section 25 cm2 is placed in a three dimensional coordinate system as shown in the figure. The electric field in the region is given by `vecE = 20 xhati` where E is NC−1 and x is in metres. Find
(i) Net flux through the cylinder.
(ii) Charge enclosed by the cylinder.
Solution
(i) Given,
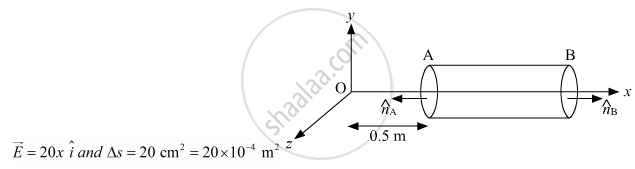
As the electric field is only along the x-axis, so, flux will pass only through the cross-section of cylinder.
Magnitude of electric field at A,
`E_A = 20 xx 0.5 = 10 N C^-1`
Magnitude of lelectic field at cross - section B
EB = 20 × 1= 20 NC-1
The corresponding eletric fluxes are
`phi_A = vecE.Δvecs = 10 xx 20 xx 10^-4 xx cos 180° = - 0.02 N m^2 C^-1`
`phi_B = vecE.Δvecs = 20 xx 20 xx 10^-4 xx cos0° = 0.04 N m^2 C^-1`
So, the net flux through the cylinder,
`phi = phi _A + phi_B = - 0.02 + 0.04 = 0.02 N m^2 C^-1 xx 10^12C`
(ii) Using Gauss’s law:
`oint vecE. vecds = q/in_0 => 0.02 = q/(8.85 xx 10^-12) => q =8.85 xx 0.02 xx 10^-12 = 0.177 xx 10^12 C`
APPEARS IN
RELATED QUESTIONS
Show that if we connect the smaller and the outer sphere by a wire, the charge q on the former will always flow to the latter, independent of how large the charge Q is.
Consider a system of n charges q1, q2, ... qn with position vectors `vecr_1,vecr_2,vecr_3,...... vecr_n`relative to some origin 'O'. Deduce the expression for the net electric field`vec E` at a point P with position vector `vecr_p,`due to this system of charges.
The charge on a proton is +1.6 × 10−19 C and that on an electron is −1.6 × 10−19 C. Does it mean that the electron has 3.2 × 10−19 C less charge than the proton?
When the separation between two charges is increased, the electric potential energy of the charges
Consider a uniformly charged ring of radius R. Find the point on the axis where the electric field is maximum.
A particle of mass m and charge q is thrown at a speed u against a uniform electric field E. How much distance will it travel before coming to momentary rest ?
12 J of work has to be done against an existing electric field to take a charge of 0.01 C from A to B. How much is the potential difference VB − VA?
An electric field \[\vec{E} = \vec{i}\] Ax exists in space, where A = 10 V m−2. Take the potential at (10 m, 20 m) to be zero. Find the potential at the origin.
Two similar spheres having +Q and -Q charges are kept at a certain distance. F force acts between the two. If at the middle of two spheres, another similar sphere having +Q charge is kept, then it experiences a force in magnitude and direction as ______.
Five charges, q each are placed at the corners of a regular pentagon of side ‘a’ (Figure).
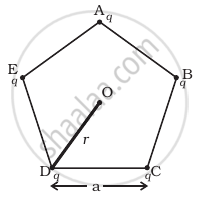
(a) (i) What will be the electric field at O, the centre of the pentagon?
(ii) What will be the electric field at O if the charge from one of the corners (say A) is removed?
(iii) What will be the electric field at O if the charge q at A is replaced by –q?
(b) How would your answer to (a) be affected if pentagon is replaced by n-sided regular polygon with charge q at each of its corners?
