Advertisements
Advertisements
Question
A horizontal overhead power line carries a current of 90 A in east to west direction. What is the magnitude and direction of the magnetic field due to the current 1.5 m below the line?
Solution
Current in the power line, I = 90 A
Point is located below the power line at distance, r = 1.5 m
Hence, magnetic field at that point is given by the relation,
B =
Where,
μ0 = Permeability of free space = 4π × 10–7 T m A–1
B =
= 1.2 × 10–5 T
The current is flowing from East to West. The point is below the power line. Hence, according to Maxwell’s right-hand thumb rule, the direction of the magnetic field is towards the South.
APPEARS IN
RELATED QUESTIONS
(a) Write the expression for the magnetic force acting on a charged particle moving with velocity v in the presence of magnetic field B.
A circular coil of wire consisting of 100 turns, each of radius 8.0 cm carries a current of 0.40 A. What is the magnitude of the magnetic field B at the centre of the coil?
A straight wire carrying an electric current is placed along the axis of a uniformly charged ring. Will there be a magnetic force on the wire if the ring starts rotating about the wire? If yes, in which direction?
A straight horizontal wire of mass 10 mg and length 1.0 m carries a current of 2.0 A. What minimum magnetic field B should be applied in the region, so that the magnetic force on the wire may balance its weight?
Write the expression for the Lorentz force F in vector form.
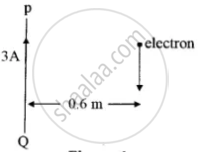
PQ is a long straight conductor carrying a current of 3A as shown in Figure below. An electron moves with a velocity of 2 x 107 ms-1 parallel to it. Find the force acting on the electron.
A charged particle is in motion having initial velocity
For a circular coil of radius R and N turns carrying current I, the magnitude of the magnetic field at a point on its axis at a distance x from its centre is given by,
B =
(a) Show that this reduces to the familiar result for field at the centre of the coil.
(b) Consider two parallel co-axial circular coils of equal radius R, and number of turns N, carrying equal currents in the same direction, and separated by a distance R. Show that the field on the axis around the mid-point between the coils is uniform over a distance that is small as compared to R, and is given by, B =
[Such an arrangement to produce a nearly uniform magnetic field over a small region is known as Helmholtz coils.]
A magnetic field set up using Helmholtz coils is uniform in a small region and has a magnitude of 0.75 T. In the same region, a uniform electrostatic field is maintained in a direction normal to the common axis of the coils. A narrow beam of (single species) charged particles all accelerated through 15 kV enters this region in a direction perpendicular to both the axis of the coils and the electrostatic field. If the beam remains undeflected when the electrostatic field is 9.0 × 10–5 V m–1, make a simple guess as to what the beam contains. Why is the answer not unique?
A solenoid 60 cm long and of radius 4.0 cm has 3 layers of windings of 300 turns each. A 2.0 cm long wire of mass 2.5 g lies inside the solenoid (near its centre) normal to its axis; both the wire and the axis of the solenoid are in the horizontal plane. The wire is connected through two leads parallel to the axis of the solenoid to an external battery which supplies a current of 6.0 A in the wire. What value of current (with appropriate sense of circulation) in the windings of the solenoid can support the weight of the wire? (g = 9.8 m s–2)
The correct plot of the magnitude of magnetic field
Which one of the following is a correct statement about magnetic forces?
In the product
=
For q = 1 and
What will be the complete expression for
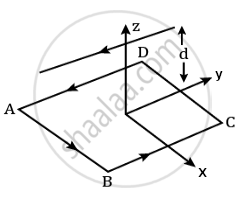
Figure shows a square loop. 20 cm on each side in the x-y plane with its centre at the origin. The loop carries a current of 7 A. Above it at y = 0, z = 12 cm is an infinitely long wire parallel to the x axis carrying a current of 10 A. The net force on the loop is ______ × 10-4 N.
A unit vector is represented as
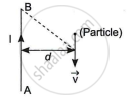
A long straight wire AB carries a current I. A particle (mass m and charge q) moves with a velocity
Lorentz force in vector form is ______.
