Advertisements
Advertisements
Question
A mercury lamp is a convenient source for studying frequency dependence of photoelectric emission, since it gives a number of spectral lines ranging from the UV to the red end of the visible spectrum. In our experiment with rubidium photo-cell, the following lines from a mercury source were used:
λ1 = 3650 Å, λ2 = 4047 Å, λ3 = 4358 Å, λ4 = 5461 Å, λ5 = 6907 Å,
The stopping voltages, respectively, were measured to be:
V01 = 1.28 V, V02 = 0.95 V, V03 = 0.74 V, V04 = 0.16 V, V05 = 0 V
Determine the value of Planck’s constant h, the threshold frequency and work function for the material.
[Note: You will notice that to get h from the data, you will need to know e (which you can take to be 1.6 × 10−19 C). Experiments of this kind on Na, Li, K, etc. were performed by Millikan, who, using his own value of e (from the oil-drop experiment) confirmed Einstein’s photoelectric equation and at the same time gave an independent estimate of the value of h.]
Solution
Einstein’s photoelectric equation is given as:
eV0 = hv − `phi_0`
`"V"_0 = "h"/"e" "v" - phi_0/"e"` .............(1)
Where,
V0 = Stopping potential
h = Planck’s constant
e = Charge on an electron
v = Frequency of radiation
`phi_0` = Work function of a material
It can be concluded from equation (1) that potential V0 is directly proportional to frequency v.
Frequency is also given by the relation:
`"v" = "Speed of light (c)"/"Wavelenght (λ)"`
This relation can be used to obtain the frequencies of the various lines of the given wavelengths.
`"v"_1 = "c"/lambda_1 = (3 xx 10^8)/(3650 xx 10^(-10)) = 8.219 xx 10^14 "Hz"`
`"v"_2 = "c"/lambda_2 = (3 xx10^8)/(4047 xx 10^(-10)) = 7.412 xx 10^14 "Hz"`
`"v"_3 = "c"/lambda_3 = (3 xx 10^8)/(4358 xx 10^(-10)) = 6.884 xx 10^14 "Hz"`
`"v"_4 = "c"/lambda_4 = (3 xx 10^8)/(5461 xx 10^(-10)) = 5.493 xx 10^14 "Hz"`
`"v"_5 = "c"/lambda_5 = (3xx10^8)/(6907 xx 10^(-10)) = 4.343 xx 10^14 "Hz"`
The given quantities can be listed in tabular form as:
| Frequency × 1014 Hz | 8.219 | 7.412 | 6.884 | 5.493 | 4.343 |
| Stopping potential V0 | 1.28 | 0.95 | 0.74 | 0.16 | 0 |
The following figure shows a graph between νand V0.
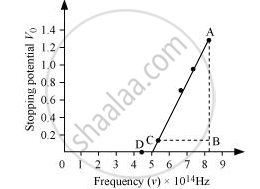
It can be observed that the obtained curve is a straight line. It intersects the ν-axis at 5 × 1014 Hz, which is the threshold frequency (v0) of the material. Point D corresponds to a frequency less than the threshold frequency. Hence, there is no photoelectric emission for the λ5 line, and therefore, no stopping voltage is required to stop the current.
Slope of the straight line = `"AB"/"CB" = (1.28 - 0.16)/((8.214 - 5.493) xx 10^14)`
From equation (1), the slope `"h"/"e"` can be written as:
`"h"/"e" = (1.28 - 0.16)/((8.214 - 5.493) xx 10^14)`
∴ `"h" = (1.12 xx 1.6 xx 10^(-19))/(2.726 xx 10^(14))`
= 6.573 × 10−34 Js
The work function of the metal is given as:
`phi_0` = hv0
= 6.573 × 10−34 × 5 × 1014
= 3.286 × 10−19 J
= `(3.286 xx 10^(-19))/(1.6 xx 1^(-18))`
= 2.054 eV
APPEARS IN
RELATED QUESTIONS
Define the term 'intensity of radiation' in terms of photon picture of light.
A hot body is placed in a closed room maintained at a lower temperature. Is the number of photons in the room increasing?
It is found that photosynthesis starts in certain plants when exposed to sunlight, but it does not start if the plants are exposed only to infrared light. Explain.
When the intensity of a light source in increased,
(a) the number of photons emitted by the source in unit time increases
(b) the total energy of the photons emitted per unit time increases
(c) more energetic photons are emitted
(d) faster photons are emitted
A parallel beam of monochromatic light of wavelength 663 nm is incident on a totally reflecting plane mirror. The angle of incidence is 60° and the number of photons striking the mirror per second is 1.0 × 1019. Calculate the force exerted by the light beam on the mirror.
(Use h = 6.63 × 10-34J-s = 4.14 × 10-15 eV-s, c = 3 × 108 m/s and me = 9.1 × 10-31kg)
A sphere of radius 1.00 cm is placed in the path of a parallel beam of light of large aperture. The intensity of the light is 0.5 W cm−2. If the sphere completely absorbs the radiation falling on it, Show that the force on the sphere due to the light falling on it is the same even if the sphere is not perfectly absorbing.
Show that it is not possible for a photon to be completely absorbed by a free electron.
The work function of a metal is 2.5 × 10−19 J. (a) Find the threshold frequency for photoelectric emission. (b) If the metal is exposed to a light beam of frequency 6.0 × 1014 Hz, what will be the stopping potential?
(Use h = 6.63 × 10-34J-s = 4.14 × 10-15 eV-s, c = 3 × 108 m/s and me = 9.1 × 10-31kg)
When a metal plate is exposed to a monochromatic beam of light of wavelength 400 nm, a negative potential of 1.1 V is needed to stop the photo current. Find the threshold wavelength for the metal.
(Use h = 6.63 × 10-34J-s = 4.14 × 10-15 eV-s, c = 3 × 108 m/s and me = 9.1 × 10-31kg)
The electric field associated with a monochromatic beam is 1.2 × 1015 times per second. Find the maximum kinetic energy of the photoelectrons when this light falls on a metal surface whose work function is 2.0 eV.
(Use h = 6.63 × 10-34J-s = 4.14 × 10-15 eV-s, c = 3 × 108 m/s and me = 9.1 × 10-31kg)
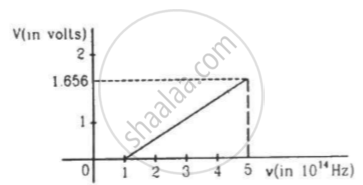
The figure is the plot of stopping potential versus the frequency of the light used in an experiment on photoelectric effect. Find (a) the ratio h/e and (b) the work function.
Define the term: stopping potential in the photoelectric effect.
Consider a thin target (10–2 cm square, 10–3 m thickness) of sodium, which produces a photocurrent of 100 µA when a light of intensity 100W/m2 (λ = 660 nm) falls on it. Find the probability that a photoelectron is produced when a photons strikes a sodium atom. [Take density of Na = 0.97 kg/m3].
The graph shows the variation of photocurrent for a photosensitive metal
- What does X and A on the horizontal axis represent?
- Draw this graph for three different values of frequencies of incident radiation ʋ1, ʋ2 and ʋ3 (ʋ3 > ʋ2 > ʋ1) for the same intensity.
- Draw this graph for three different values of intensities of incident radiation I1, I2 and I3 (I3 > I2 > I1) having the same frequency.
If photons of ultraviolet light of energy 12 eV are incident on a metal surface of work function of 4 eV, then the stopping potential (in eV) will be :
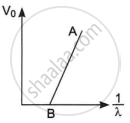
The figure shows a plot of stopping potential (V0) versus `1/lambda`, where λ is the wavelength of the radiation causing photoelectric emission from a surface. The slope of the line is equal to ______.
A metallic plate exposed to white light emits electrons. For which of the following colours of light, the stopping potential will be maximum?
What is the effect of threshold frequency and stopping potential on increasing the frequency of the incident beam of light? Justify your answer.
