Advertisements
Advertisements
Question
A metallic cylinder has a radius of 3 cm and a height of 5 cm. It is made of metal A. To reduce its weight, a conical hole is drilled in the cylinder, as shown and it is completely filled with a lighter metal B. The conical hole has a radius of `3/2` cm and its depth is `8/9` cm. Calculate the ratio of the volume of the metal A to the volume of metal B in the solid.
Solution
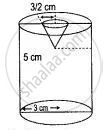
Volume of metal A = Volume of the cylinder - Volume of the cone
= `π (3)^2 x 5 - 1/3 π (3/2)^2 8/9`
= `π ( 45 - 2/3)`
= `133/3` π cm3
Volume of metal B = Volume of the conical cavity
= `1/3 xx (3/2)^2 . 8/9 = 2/3 π`
Hence, ratio of the volume of the metal A to the volume of the metal B.
= `(133/3 π)/(2/3 π) = 133/2`
= 66.5: 1.
APPEARS IN
RELATED QUESTIONS
Curved surface area of a cone is 308 cm2 and its slant height is 14 cm. Find
- radius of the base and
- total surface area of the cone.
`["Assume "pi =22/7]`
Find the total surface area of a cone, if its slant height is 21 m and diameter of its base is 24 m.
The area of the curved surface of a cone is 60 cm2. If the slant height of the cone be 8 cm, find the radius of the base?
Find the volume of a right circular cone with:
radius 3.5 cm, height 12 cm
Find the volume of a right circular cone with:
height 21 cm and slant height 28 cm.
The ratio of volumes of two cones is 4 : 5 and the ratio of the radii of their bases is 2:3. Find the ratio of their vertical heights.
Find the volume of the largest right circular cone that can be fitted in a cube whose edge is 14 cm.
Monica has a piece of Canvas whose area is 551 m2. She uses it to have a conical tent made, with a base radius of 7m. Assuming that all the stitching margins and wastage incurred while cutting, amounts to approximately 1 m2. Find the volume of the tent that can be made with it.
Find the volume of a cone whose slant height is 17 cm and radius of base is 8 cm.
The diameter of two cones are equal. If their slant heights are in the ratio 5 : 4, find the ratio of their curved surface areas.
A solid sphere and a solid hemi-sphere have the same total surface area. Find the ratio between their volumes.
A solid metallic hemisphere of diameter 28 cm is melted and recast into a number of identical solid cones, each of diameter 14 cm and height 8 cm. Find the number of cones so formed.
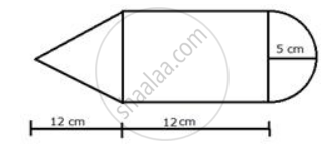
The horizontal cross-section of a water tank is in the shape of a rectangle with semi-circle at one end, as shown in the following figure. The water is 2.4 metres deep in the tank. Calculate the volume of water in the tank in gallons.

Find the height of the cone whose base radius is 5 cm and volume is 75π cm3.
The given figure shows the cross-section of a cone, a cylinder and a hemisphere all with the same diameter 10 cm and the other dimensions are as shown. Calculate: the total volume of the solid.
A cone and a hemisphere have equal bases and equal volumes. Find the ratio of their heights.
The curved surface area of a right circular cone of height 15 cm and base diameter 16 cm is
How many square metres of canvas is required for a conical tent whose height is 3.5 m and the radius of the base is 12 m?
A cloth having an area of 165 m2 is shaped into the form of a conical tent of radius 5 m. Find the volume of the cone.
