Advertisements
Advertisements
Question
A rhombus shaped field has green grass for 18 cows to graze. If each side of the rhombus is 30 m and its longer diagonal is 48 m, how much area of grass field will each cow be getting?
Solution
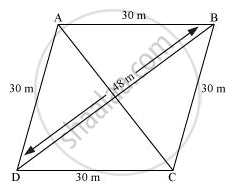
Let ABCD be a rhombus-shaped field.
For ΔBCD,
Semi-perimeter,
`s=(48+30+30)/2 = 54 m`
By Heron's Formula,
`"Area of triangle "=sqrt(s(s-a)(s-b)(s-c))`
`therefore" Area of ΔBCD "=[sqrt(54(54-48)(54-30)(54-30))]m^2`
`=sqrt(54(6)(24)(24))`
= 3 x 6 x 24
= 432 m2
Area of field = 2 × Area of ΔBCD
= (2 × 432) m2 = 864 m2
Area for grazing for 1 cow = 864/18 = 48 m2
Each cow will get 48 m2 area of grass field.
APPEARS IN
RELATED QUESTIONS
Radha made a picture of an aeroplane with coloured papers as shown in the given figure. Find the total area of the paper used.
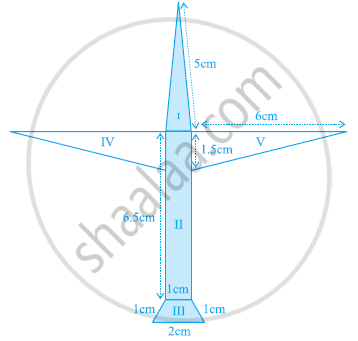
Area of PQRS = Area of PQR + Area of ΔPQS = (6+9.166)𝑐𝑚2=15.166𝑐𝑚2
Find the area of a quadrilateral ABCD in which AD = 24 cm, ∠BAD = 90° and BCD forms an equilateral triangle whose each side is equal to 26 cm. (Take √3 = 1.73)
The perimeter of a triangullar field is 144 m and the ratio of the sides is 3 : 4 : 5. Find the area of the field.
If each side of a triangle is doubled, the find percentage increase in its area.
The sides of a triangular field are 325 m, 300 m and 125 m. Its area is
The sides of a triangle are 50 cm, 78 cm and 112 cm. The smallest altitude is
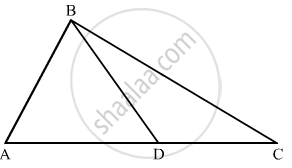
In the given figure, the ratio AD to DC is 3 to 2. If the area of Δ ABC is 40 cm2, what is the area of Δ BDC?
A square and an equilateral triangle have equal perimeters. If the diagonal of the square is \[12\sqrt{2}\] cm, then area of the triangle is
The area of an equilateral triangle with side `2sqrt(3)` cm is ______.
