Advertisements
Advertisements
Question
A rod of length L has a total charge Q distributed uniformly along its length. It is bent in the shape of a semicircle. Find the magnitude of the electric field at the centre of curvature of the semicircle.
Solution
Consider an element of angular width dθ, as shown in the figure.
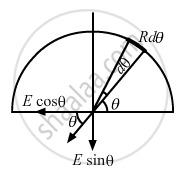
\[dq = \frac{Q}{L}Rd\theta\]
The net electric field has a vertical component only.
\[\text{So}, E_{net} = \int dE \sin\theta\]
\[ = \frac{1}{4\pi \epsilon_0}\frac{Q}{L} \int\limits_0^\pi \frac{Rd\theta}{R^2}\sin\theta\]
\[= \frac{- 1}{4\pi \epsilon_0}\frac{Q}{LR} \left[ \cos\theta \right]_0^\pi \]
\[ = \frac{2Q}{4\pi \epsilon_0 LR}\]
\[ = \frac{Q}{2 \epsilon_0 L^2} \left( \because \pi R = L \right)\]
APPEARS IN
RELATED QUESTIONS
Write the expression for the displacement current in terms of the rate of change of electric flux.
A point charge is brought inside an electric field. The electric field at a nearby point
(a) will increase if the charge is positive
(b) will decrease if the charge is negative
(c) may increase if the charge is positive
(d) may decrease if the charge is negative
A proton and an electron are placed in a uniform electric field.
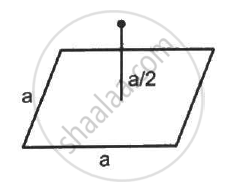
A charge Q is placed at a distance a/2 above the centre of a horizontal, square surface of edge a as shown in the following figure . Find the flux of the electric field through the square surface.
Two large conducting plates are placed parallel to each other with a separation of 2⋅00 cm between them. An electron starting from rest near one of the plates reaches the other plate in 2⋅00 microseconds. Find the surface charge density on the inner surfaces.
A positive charge q is placed in front of a conducting solid cube at a distance d from its centre. Find the electric field at the centre of the cube to the charges appearing on its surface.
Choose the correct option.
Two point charges of +5 μC are so placed that they experience a force of 8.0 × 10-3N. They are then moved apart so that the force is now 2.0 × 10-3N. The distance between them is now
Answer the following question.
What is the magnitude of the charge on an electron?
Two small conducting spheres of equal radius have charges +10 µC and -20 µC respectively and placed at a distance R from each other experience force F1· If they are brought in contact and separated to the same distance, they experience force F2. The ratio of F1 to F2 is ____________.
Conductors are materials that allow ______.
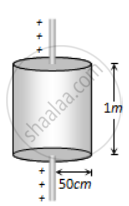
Electric charge is uniformly distributed along a long straight wire of radius 1 mm. The charge per cm length of the wire is Q coulomb. Another cylindrical surface of radius 50 cm and length 1 m symmetrically enclose the wire as shown in the figure. The total electric flux passing through the cylindrical surface is ______.
When a glass rod is rubbed with silk, it ______.
A metallic spherical shell has an inner radius R1 and outer radius R2. A charge Q is placed at the centre of the spherical cavity. What will be surface charge density on (i) the inner surface, and (ii) the outer surface?
Given below are two statements:
- Statement I: The electric force changes the speed of the charged particle and hence changes its kinetic energy; whereas the magnetic force does not change the kinetic energy of the charged particle.
- Statement II: The electric force accelerates the positively charged particle perpendicular to the direction of the electric field. The magnetic force accelerates the moving charged particle along the direction of the magnetic field.
In light of the above statements, choose the most appropriate answer from the options given below.
Two identical metallic spheres A and B when placed at certain distance in air repel each other with a force of F. Another identical uncharged sphere C is first placed in contact with A and then in contact with B and finally placed at midpoint between spheres A and B. The force experienced by sphere C will be:
The electrostatic potential inside a charged spherical ball is given by `Phi = ar^2 + b`, where r is the distance from the centre a, and b are constants. Then the charge density inside the ball is ______.
A charge of magnitude 3e and mass 2m is moving in an electric field E. The acceleration imparted to the charge is ______.
