Advertisements
Online Mock Tests
Chapters
2: Kinetic Theory of Gases
3: Calorimetry
4: Laws of Thermodynamics
5: Specific Heat Capacities of Gases
6: Heat Transfer
▶ 7: Electric Field and Potential
8: Gauss’s Law
9: Capacitors
10: Electric Current in Conductors
11: Thermal and Chemical Effects of Current
12: Magnetic Field
13: Magnetic Field due to a Current
14: Permanent Magnets
15: Magnetic Properties of Matter
16: Electromagnetic Induction
17: Alternating Current
18: Electromagnetic Waves
19: Electric Current through Gases
20: Photoelectric Effect and Wave-Particle Duality
21: Bohr’s Model and Physics of Atom
22: X-rays
23: Semiconductors and Semiconductor Devices
24: The Nucleus
25: The Special Theory of Relativity
![HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 chapter 7 - Electric Field and Potential HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 chapter 7 - Electric Field and Potential - Shaalaa.com](/images/9788177092325-concepts-of-physics-vol-2-english-class-11-and-12_6:cd4e4bfcb8474a60871d8e5659ec4eb9.jpg)
Advertisements
Solutions for Chapter 7: Electric Field and Potential
Below listed, you can find solutions for Chapter 7 of CBSE, Karnataka Board PUC HC Verma for Concepts of Physics Vol. 2 [English] Class 11 and 12.
HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 7 Electric Field and Potential Short Answers [Page 119]
The charge on a proton is +1.6 × 10−19 C and that on an electron is −1.6 × 10−19 C. Does it mean that the electron has 3.2 × 10−19 C less charge than the proton?
Is there any lower limit to the electric force between two particles placed at a separation of 1 cm?
Consider two particles A and B of equal charges placed at some distance. Particle A is slightly displaced towards B. Does the force on B increase as soon as particle A is displaced? Does the force on particle A increase as soon as it is displaced?
Can a gravitational field be added vectorially to an electric field to get a total field?
Why does a phonograph record attract dust particles just after it is cleaned?
Does the force on a charge due to another charge depend on the charges present nearby?
In some old texts it is mentioned that 4π lines of force originate from each unit positive charge. Comment on the statement in view of the fact that 4π is not an integer.
Can two equipotential surfaces cut each other?
If a charge is placed at rest in an electric field, will its path be along a line of force? Discuss the situation when the lines of force are straight and when they are curved.
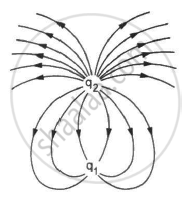
Consider the situation shown in the figure. What are the signs of q1 and q2? If the lines are drawn in proportion to the charges, what is the ratio q1/q2?
A point charge is taken from a point A to a point B in an electric field. Does the work done by the electric field depend on the path of the charge?
It is said that the separation between the two charges forming an electric dipole should be small. In comparison to what should this separation be small?
The number of electrons in an insulator is of the same order as the number of electrons in a conductor. What is then the basic difference between a conductor and an insulator ?
When a charged comb is brought near a small piece of paper, it attracts the piece. Does the paper become charged when the comb is brought near it?
HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 7 Electric Field and Potential Short Answers [Pages 119 - 120]
Figure shows some of the electric field lines corresponding to an electric field. The figure suggests that

EA > EB > EC
EA = EB = EC
EA = EC > EB
EA = EC < EB
When the separation between two charges is increased, the electric potential energy of the charges
increases
decreases
remains the same
may increase or decrease
If a positive charge is shifted from a low-potential region to a high-potential region, the electric potential energy
increases
decreases
remains the same
may increase of decrease
Two equal positive charges are kept at points A and B. The electric potential at the points between A and B (excluding these points) is situated while moving from A to B. The potential
continuously increases
continuously decreases
increases then decreases
decreases then increases
The electric field at the origin is along the positive x-axis. A small circle is drawn with the centre at the origin, cutting the axes at points A, B, C and D with coordinates (a, 0), (0, a), (−a, 0), (0, −a), respectively. Out of the points on the periphery of the circle, the potential is minimum at
A
B
C
D
If a body is charged by rubbing it, its weight
remains precisely constant
increases slightly
decreases slightly
may increase slightly or may decrease slightly
An electric dipole is placed in a uniform electric field. The net electric force on the dipole
is always zero
depends on the orientation of the dipole
can never be zero
depends on the strength of the dipole
Consider the situation in the figure. The work done in taking a point charge from P to Ais WA, from P to B is WB and from P to C is WC.
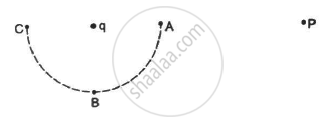
WA < WB < WC
WA > WB > WC
WA = WB = WC
None of these
A point charge q is rotated along a circle in an electric field generated by another point charge Q. The work done by the electric field on the rotating charge in one complete revolution is
zero
positive
negative
zero if the charge Q is at the centre, otherwise non-zero
HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 7 Electric Field and Potential MCQ [Page 120]
Mark out the correct options.
The total charge of the universe is constant.
The total positive charge of the universe is constant.
The total negative charge of the universe is constant.
The total number of charged particles in the universe is constant.
A point charge is brought inside an electric field. The electric field at a nearby point
(a) will increase if the charge is positive
(b) will decrease if the charge is negative
(c) may increase if the charge is positive
(d) may decrease if the charge is negative
The electric field and the electric potential at a point are E and V, respectively.
If E = 0, V must be zero.
If V = 0, E must be zero.
If E ≠ 0, V cannot be zero.
If V ≠0, E cannot be zero.
None of the above.
Electric potential decreases uniformly from 120 V to 80 V, as one moves on the x-axis from x = −1 cm to x = +1 cm. The electric field at the origin
(a) must be equal to 20 Vcm−1
(b) may be equal to 20 Vcm−1
(c) may be greater than 20 Vcm−1
(d) may be less than 20 Vcm−1
Which of the following quantities does not depend on the choice of zero potential or zero potential energy?
Potential at a point
Potential difference between two points
Potential energy of a two-charge system
None of these
An electric dipole is placed in an electric field generated by a point charge.
The net electric force on the dipole must be zero.
The net electric force on the dipole may be zero.
The torque on the dipole due to the field must be zero.
The torque on the dipole due to the field may be zero.
A proton and an electron are placed in a uniform electric field.
The electric forces acting on them will be equal.
The magnitudes of the forces will be equal.
Their accelerations will be equal.
The magnitudes of their accelerations will be equal.
The electric field in a region is directed outward and is proportional to the distance rfrom the origin. Taking the electric potential at the origin to be zero,
it is uniform in the region
it is proportional to r
it is proportional to r2
it increases as one moves away from the origin
HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 7 Electric Field and Potential Exercises [Pages 121 - 124]
Find the dimensional formula of ε0.
A charge of 1.0 C is placed at the top of your college building and another equal charge at the top of your house. Take the separation between the two charges to be 2.0 km. Find the force exerted by the charges on each other. How many times your weight is this force?
At what separation should two equal charges, 1.0 C each, be placed, so that the force between them equals the weight of a 50 kg person?
Two equal charges are placed at a separation of 1.0 m. What should be the magnitude of the charges, so that the force between them equals the weight of a 50 kg person?
Find the electric force between two protons separated by a distance of 1 fermi (1 fermi = 10−15 m). The protons in a nucleus remain at a separation of this order.
Two charges 2.0 × 10−6 C and 1.0 × 10−6 C are placed at a separation of 10 cm. Where should a third charge be placed, such that it experiences no net force due to these charges?
Suppose the second charge in the previous problem is −1.0 × 10−6 C. Locate the position where a third charge will not experience a net force.
Two charged particles are placed 1.0 cm apart. What is the minimum possible magnitude of the electric force acting on each charge?
Estimate the number of electrons in 100 g of water. How much is the total negative charge on these electrons?
Suppose all the electrons of 100 g water are lumped together to form a negatively-charged particle and all the nuclei are lumped together to form a positively-charged particle. If these two particles are placed 10.0 cm away from each other, find the force of attraction between them. Compare it with your weight.
Consider a gold nucleus to be a sphere of radius 6.9 fermi in which protons and neutrons are distributed. Find the force of repulsion between two protons situated at largest separation. Why do these protons not fly apart under this repulsion?
Two insulating small spheres are rubbed against each other and placed 1 cm apart. If they attract each other with a force of 0.1 N, how many electrons were transferred from one sphere to the other during rubbing?
NaCl molecule is bound due to the electric force between the sodium and the chlorine ions when one electron of sodium is transferred to chlorine. Taking the separation between the ions to be 2.75 × 10−8 cm, find the force of attraction between them. State the assumptions (if any) that you have made.
Find the ratio of the electrical and gravitational forces between two protons.
Suppose an attractive nuclear force acts between two protons which may be written as F=Ce−kr/r2. Write down the dimensional formulae and appropriate SI units of C and k.
Suppose an attractive nuclear force acts between two protons which may be written as F=Ce−kr/r2. Suppose that k = 1 fermi−1 and that the repulsive electric force between the protons is just balanced by the attractive nuclear force when the separation is 5 fermi. Find the value of C.
Three equal charges, 2.0 × 10−6 C each, are held at the three corners of an equilateral triangle of side 5 cm. Find the Coulomb force experienced by one of the charges due to the other two.
Four equal charges of 2.0 × 10−6 C each are fixed at the four corners of a square of side 5 cm. Find the Coulomb's force experienced by one of the charges due to the other three.
A hydrogen atom contains one proton and one electron. It may be assumed that the electron revolves in a circle of radius 0.53 angstrom (1 angstrom = 10−10 m and is abbreviated as Å ) with the proton at the centre. The hydrogen atom is said to be in the ground state in this case. Find the magnitude of the electric force between the proton and the electron of a hydrogen atom in its ground state.
Find the speed of the electron in the ground state of a hydrogen atom. The description of ground state is given in the previous problem.
Ten positively-charged particles are kept fixed on the x-axis at points x = 10 cm, 20 cm, 30 cm, ...., 100 cm. the first particle has a charge 1.0 × 10−8 C, the second 8 × 10−8 C, the third 27 × 10−8 C and so on. The tenth particle has a charge 1000 × 10−8 C. Find the magnitude of the electric force acting on a 1 C charge placed at the origin.
Two charged particles with charge 2.0 × 10−8 C each are joined by an insulating string of length 1 m and the system is kept on a smooth horizontal table. Find the tension in the string.
Two identical balls, each with a charge of 2.00 × 10−7 C and a mass of 100 g, are suspended from a common point by two insulating strings, each 50 cm long. The balls are held at a separation 5.0 cm apart and then released. Find.
(a) the electric force on one of the charged balls
(b) the components of the resultant force on it along and perpendicular to the string
(c) the tension in the string
(d) the acceleration of one of the balls. Answers are to be obtained only for the instant just after the release.
Two identical pith balls are charged by rubbing one against the other. They are suspended from a horizontal rod through two strings of length 20 cm each, the separation between the suspension points being 5 cm. In equilibrium, the separation between the balls is 3 cm. Find the mass of each ball and the tension in the strings. The charge on each ball has a magnitude 2.0 × 10−8 C.
Two small spheres, each with a mass of 20 g, are suspended from a common point by two insulating strings of length 40 cm each. The spheres are identically charged and the separation between the balls at equilibrium is found to be 4 cm. Find the charge on each sphere.
Two identical pith balls, each carrying a charge q, are suspended from a common point by two strings of equal length l. Find the mass of each ball if the angle between the strings is 2θ in equilibrium.
A particle with a charge of 2.0 × 10−4 C is placed directly below and at a separation of 10 cm from the bob of a simple pendulum at rest. The mass of the bob is 100 g. What charge should the bob be given so that the string becomes loose?
Two particles A and B with charges q and 2q, respectively, are placed on a smooth table with a separation d. A third particle C is to be clamped on the table in such a way that the particles A and B remain at rest on the table under electrical forces. What should be the charge on C and where should it be clamped?
Two identically-charged particles are fastened to the two ends of a spring of spring constant 100 N m−1 and natural length 10 cm. The system rests on a smooth horizontal table. If the charge on each particle is 2.0 × 10−8 C, find the extension in the length of the spring. Assume that the extension is small as compared to the natural length. Justify this assumption after you solve the problem.
A particle A with a charge of 2.0 × 10−6 C is held fixed on a horizontal table. A second charged particle of mass 80 g stays in equilibrium on the table at a distance of 10 cm from the first charge. The coefficient of friction between the table and this second particle is μ = 0.2. Find the range within which the charge of this second particle may lie.
A particle A with a charge of 2.0 × 10−6 C and a mass of 100 g is placed at the bottom of a smooth inclined plane of inclination 30°. Where should another particle B, with the same charge and mass, be placed on the incline so that it may remain in equilibrium?
Two particles A and B, each with a charge Q, are placed a distance d apart. Where should a particle of charge q be placed on the perpendicular bisector of AB, so that it experiences maximum force? What is the magnitude of this maximum force?
Two particles A and B, each carrying a charge Q, are held fixed with a separation dbetween them. A particle C of mass m and charge q is kept at the middle point of the line AB. If it is displaced through a distance x perpendicular to AB, what would be the electric force experienced by it?
Two particles A and B, each carrying a charge Q, are held fixed with a separation dbetween them. A particle C of mass m and charge q is kept at the middle point of the line AB. Assuming x<<d, show that this force is proportional to x.
Two particles A and B, each carrying a charge Q, are held fixed with a separation dbetween them. A particle C of mass m and charge q is kept at the middle point of the line AB. Under what conditions will the particle C execute simple harmonic motion if it is released after such a small displacement? Find the time period of the oscillations if these conditions are satisfied.
Repeat the previous problem if the particle C is displaced through a distance x along the line AB.
The electric force experienced by a charge of 1.0 × 10−6 C is 1.5 × 10−3 N. Find the magnitude of the electric field at the position of the charge.
Two particles A and B possessing charges of +2.00 × 10−6 C and of −4.00 × 10−6 C, respectively, are held fixed at a separation of 20.0 cm. Locate the points (s) on the line AB, where (a) the electric field is zero (b) the electric potential is zero.
A point charge produces an electric field of magnitude 5.0 NC−1 at a distance of 40 cm from it. What is the magnitude of the charge?
A water particle of mass 10.0 mg and with a charge of 1.50 × 10−6 C stays suspended in a room. What is the magnitude of electric field in the room? What is its direction ?
Three identical charges, each with a value of 1.0 × 10−8 C, are placed at the corners of an equilateral triangle of side 20 cm. Find the electric field and potential at the centre of the triangle.
A positive charge Q is distributed uniformly over a circular ring of radius R. A particle of mass m, and a negative charge q, is placed on its axis at a distance x from the centre. Find the force on the particle. Assuming x << R, find the time period of oscillation of the particle if it is released from there .
A rod of length L has a total charge Q distributed uniformly along its length. It is bent in the shape of a semicircle. Find the magnitude of the electric field at the centre of curvature of the semicircle.
A 10-cm long rod carries a charge of +50 μC distributed uniformly along its length. Find the magnitude of the electric field at a point 10 cm from both ends of the rod.
Consider a uniformly charged ring of radius R. Find the point on the axis where the electric field is maximum.
A wire is bent in the form of a regular hexagon and a total charge q is distributed uniformly on it. What is the electric field at the centre? You may answer this part without making any numerical calculations.
A circular wire-loop of radius a carries a total charge Q distributed uniformly over its length. A small length dL of the wire is cut off. Find the electric field at the centre due to the remaining wire.
A positive charge q is placed in front of a conducting solid cube at a distance d from its centre. Find the electric field at the centre of the cube to the charges appearing on its surface.
A pendulum bob of mass 80 mg and carrying a charge of 2 × 10−8 C is at rest in a uniform, horizontal electric field of 20 kVm−1. Find the tension in the thread.
A particle of mass m and charge q is thrown at a speed u against a uniform electric field E. How much distance will it travel before coming to momentary rest ?
A particle of mass 1 g and charge 2.5 × 10−4 C is released from rest in an electric field of 1.2 × 10 4 N C−1. Find the electric force and the force of gravity acting on this particle. Can one of these forces be neglected in comparison with the other for approximate analysis?
A particle of mass 1 g and charge 2.5 × 10−4 C is released from rest in an electric field of 1.2 × 10 4 N C−1. How long will it take for the particle to travel a distance of 40 cm?
A particle of mass 1 g and charge 2.5 × 10−4 C is released from rest in an electric field of 1.2 × 10 4 N C−1. What will be the speed of the particle after travelling this distance?
A particle of mass 1 g and charge 2.5 × 10−4 C is released from rest in an electric field of 1.2 × 10 4 N C−1. How much is the work done by the electric force on the particle during this period?
A ball of mass 100 g and with a charge of 4.9 × 10−5 C is released from rest in a region where a horizontal electric field of 2.0 × 104 N C−1 exists. (a) Find the resultant force acting on the ball. (b) What will be the path of the ball? (c) Where will the ball be at the end of 2 s?
The bob of a simple pendulum has a mass of 40 g and a positive charge of 4.0 × 10−6 C. It makes 20 oscillations in 45 s. A vertical electric field pointing upward and of magnitude 2.5 × 104 NC−1 is switched on. How much time will it now take to complete 20 oscillations?
A block of mass m with a charge q is placed on a smooth horizontal table and is connected to a wall through an unstressed spring of spring constant k, as shown in the figure. A horizontal electric field E, parallel to the spring, is switched on. Find the amplitude of the resulting SHM of the block. 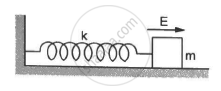
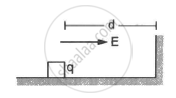
A block of mass containing a net positive charge q is placed on a smooth horizontal table which terminates in a vertical wall as shown in the figure. The distance of the block from the wall is d. A horizontal electric field E towards the right is switched on. Assuming elastic collisions (if any), find the time period of the resulting oscillatory motion. Is it a simple harmonic motion?
A uniform electric field of 10 N C−1 exists in the vertically downward direction. Find the increase in the electric potential as one goes up through a height of 50 cm.
12 J of work has to be done against an existing electric field to take a charge of 0.01 C from A to B. How much is the potential difference VB − VA?
Two equal charges, 2.0 × 10−7 C each, are held fixed at a separation of 20 cm. A third charge of equal magnitude is placed midway between the two charges. It is now moved to a point 20 cm from both the charges. How much work is done by the electric field during the process?
An electric field of 20 NC−1 exists along the x-axis in space. Calculate the potential difference VB − VA where the points A and B are
(a) A = (0, 0); B = (4 m, 2m)
(b) A = (4 m, 2 m); B = (6 m, 5 m)
(c) A = (0, 0); B = (6 m, 5 m)
Do you find any relation between the answers of parts (a), (b) and (c)?
Consider the situation of the previous problem. A charge of −2.0 × 10−4 C is moved from point A to point B. Find the change in electrical potential energy UB − UA for the cases (a), (b) and (c).
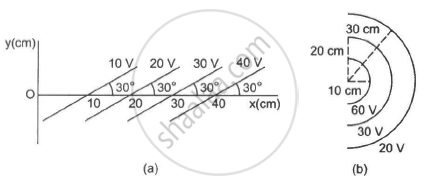
An electric field
An electric field
The electric potential existing in space is
Two charged particles, with equal charges of 2.0 × 10−5 C, are brought from infinity to within a separation of 10 cm. Find the increase in the electric potential energy during the process
Some equipotential surface is shown in the figure. What can you say about the magnitude and the direction of the electric field?
Consider a circular ring of radius r, uniformly charged with linear charge density λ. Find the electric potential at a point on the axis at a distance x from the centre of the ring. Using this expression for the potential, find the electric field at this point.
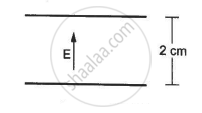
An electric field of magnitude 1000 NC−1 is produced between two parallel plates with a separation of 2.0 cm, as shown in the figure. (a) What is the potential difference between the plates? (b) With what minimum speed should an electron be projected from the lower place in the direction of the field, so that it may reach the upper plate? (c) Suppose the electron is projected from the lower place with the speed calculated in part (b). The direction of projection makes an angle of 60° with the field. Find the maximum height reached by the electron.
A uniform field of 2.0 NC−1 exists in space in the x-direction. (a) Taking the potential at the origin to be zero, write an expression for the potential at a general point (x, y, z). (b) At which point, the potential is 25 V? (c) If the potential at the origin is taken to be 100 V, what will be the expression for the potential at a general point? (d) What will be the potential at the origin if the potential at infinity is taken to be zero? Is it practical to choose the potential at infinity to be zero?
How much work has to be done in assembling three charged particles at the vertices of an equilateral triangle, as shown in the figure?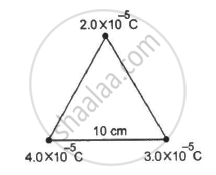
The kinetic energy of a charged particle decreases by 10 J as it moves from a point at potential 100 V to a point at potential 200 V. Find the charge on the particle.
Two identical particles, each with a charge of 2.0 × 10−4 C and mass of 10 g, are kept at a separation of 10 cm and then released. What would be the speed of the particles when the separation becomes large?
Two particles of masses 5.0 g each and opposite charges of +4.0 × 10−5 C and −4.0 × 10−5 C are released from rest with a separation of 1.0 m between them. Find the speeds of the particles when the separation is reduced to 50 cm.
A sample of HCI gas is placed in an electric field of 2.5 × 104 NC−1. The dipole moment of each HCI molecule is 3.4 × 10−30 Cm. Find the maximum torque that can act on a molecule.
Two particles A and B, of opposite charges 2.0 × 10−6 C and −2.0 × 10−6 C, are placed at a separation of 1.0 cm. Two particles A and B, of opposite charges 2.0 × 10−6 C and −2.0 × 10−6 C, are placed at a separation of 1.0 cm.
Two particles A and B, of opposite charges 2.0 × 10−6 C and −2.0 × 10−6 C, are placed at a separation of 1.0 cm. Calculate the electric field at a point on the axis of the dipole 1.0 cm away from the centre.
Two particles A and B, of opposite charges 2.0 × 10−6 C and −2.0 × 10−6 C, are placed at a separation of 1.0 cm. Calculate the electric field at a point on the perpendicular bisector of the dipole and 1.0 m away from the centre.
Three charges are arranged on the vertices of an equilateral triangle, as shown in the figure. Find the dipole moment of the combination.
Find the magnitude of the electric field at the point P in the configuration shown in the figure for d >> a.
Two particles, carrying charges −q and +q and and of mass m each, are fixed at the ends of a light rod of length a to form a dipole. The rod is clamped at an end and is placed in a uniform electric field E with the axis of the dipole along the electric field. The rod is slightly tilted and then released. Neglecting gravity, find the time period of small oscillations.
Assume that each atom in a copper wire contributes one free electron. Estimate the number of free electrons in a copper wire of mass 6.4 g (take the atomic weight of copper to be 64 g mol−1).
Solutions for 7: Electric Field and Potential
![HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 chapter 7 - Electric Field and Potential HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 chapter 7 - Electric Field and Potential - Shaalaa.com](/images/9788177092325-concepts-of-physics-vol-2-english-class-11-and-12_6:cd4e4bfcb8474a60871d8e5659ec4eb9.jpg)
HC Verma solutions for Concepts of Physics Vol. 2 [English] Class 11 and 12 chapter 7 - Electric Field and Potential
Shaalaa.com has the CBSE, Karnataka Board PUC Mathematics Concepts of Physics Vol. 2 [English] Class 11 and 12 CBSE, Karnataka Board PUC solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. HC Verma solutions for Mathematics Concepts of Physics Vol. 2 [English] Class 11 and 12 CBSE, Karnataka Board PUC 7 (Electric Field and Potential) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. HC Verma textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Concepts of Physics Vol. 2 [English] Class 11 and 12 chapter 7 Electric Field and Potential are Electric Field Due to a System of Charges, Introduction of Electric Field, Continuous Distribution of Charges, Coulomb’s Law - Force Between Two Point Charges, Basic Properties of Electric Charge, Electric Charges, Conductors and Insulators, Superposition Principle - Forces Between Multiple Charges, Physical Significance of Electric Field, Electric Field Lines, Electric Flux, Electric Dipole, Dipole in a Uniform External Field, Gauss’s Law, Applications of Gauss’s Law, Charging by Induction, Electric Field Due to a Point Charge, Uniformly Charged Infinite Plane Sheet and Uniformly Charged Thin Spherical Shell (Field Inside and Outside), Superposition Principle of Forces, Force Between Two Point Charges.
Using HC Verma Concepts of Physics Vol. 2 [English] Class 11 and 12 solutions Electric Field and Potential exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in HC Verma Solutions are essential questions that can be asked in the final exam. Maximum CBSE, Karnataka Board PUC Concepts of Physics Vol. 2 [English] Class 11 and 12 students prefer HC Verma Textbook Solutions to score more in exams.
Get the free view of Chapter 7, Electric Field and Potential Concepts of Physics Vol. 2 [English] Class 11 and 12 additional questions for Mathematics Concepts of Physics Vol. 2 [English] Class 11 and 12 CBSE, Karnataka Board PUC, and you can use Shaalaa.com to keep it handy for your exam preparation.
