Advertisements
Advertisements
Question
Two particles of masses 5.0 g each and opposite charges of +4.0 × 10−5 C and −4.0 × 10−5 C are released from rest with a separation of 1.0 m between them. Find the speeds of the particles when the separation is reduced to 50 cm.
Solution
Given:
Magnitude of charges, q = 4.0 × 10−5 C
Initial separation between charges, r = 1 m
Initial speed = 0; so, initial K.E. = 0
Mass of the particles, m = 5.0 g =0.005 kg
Let the required velocity of each particle be v.
By the law of conservation of energy,
Initial P.E. + Initial K.E. = Final P.E. + Final K.E.
\[\frac{1}{4\pi \epsilon_0}\frac{q_1 q_2}{r} = 2 \times \frac{1}{2}m v^2 + \frac{1}{4\pi \epsilon_0}\frac{q_1 q_2}{r/2}\]
\[ \Rightarrow \frac{- 1}{4\pi \epsilon_0}\frac{q^2}{r} = m v^2 - \frac{2}{4\pi \epsilon_0}\frac{q^2}{r}\]
\[ \Rightarrow m v^2 = \frac{1}{4\pi \epsilon_0}\frac{q^2}{r}\]
\[ \Rightarrow v = \sqrt{\frac{1}{4\pi \epsilon_0 m}\frac{q^2}{r}}\]
\[ \Rightarrow v = \sqrt{\frac{9 \times {10}^{- 9} \times \left( 4 \times {10}^{- 5} \right)^2}{0 . 005 \times 1}}\]
\[ \Rightarrow v = 53 . 66\] m/s
APPEARS IN
RELATED QUESTIONS
Write any two important points of similarities and differences each between Coulomb's law for the electrostatic field and Biot-Savart's law of the magnetic field ?
Plot a graph showing the variation of coulomb force (F) versus ,`(1/r^2)` where r is the distance between the two charges of each pair of charges: (1 μC, 2 μC) and (2 μC, − 3 μC). Interpret the graphs obtained.
One end of a 10 cm long silk thread is fixed to a large vertical surface of a charged non-conducting plate and the other end is fastened to a small ball of mass 10 g and a charge of 4.0× 10-6 C. In equilibrium, the thread makes an angle of 60° with the vertical. Find the surface charge density on the plate.
Find the electric force between two protons separated by a distance of 1 fermi (1 fermi = 10−15 m). The protons in a nucleus remain at a separation of this order.
Consider a gold nucleus to be a sphere of radius 6.9 fermi in which protons and neutrons are distributed. Find the force of repulsion between two protons situated at largest separation. Why do these protons not fly apart under this repulsion?
Suppose an attractive nuclear force acts between two protons which may be written as F=Ce−kr/r2. Write down the dimensional formulae and appropriate SI units of C and k.
Suppose an attractive nuclear force acts between two protons which may be written as F=Ce−kr/r2. Suppose that k = 1 fermi−1 and that the repulsive electric force between the protons is just balanced by the attractive nuclear force when the separation is 5 fermi. Find the value of C.
A hydrogen atom contains one proton and one electron. It may be assumed that the electron revolves in a circle of radius 0.53 angstrom (1 angstrom = 10−10 m and is abbreviated as Å ) with the proton at the centre. The hydrogen atom is said to be in the ground state in this case. Find the magnitude of the electric force between the proton and the electron of a hydrogen atom in its ground state.
Find the speed of the electron in the ground state of a hydrogen atom. The description of ground state is given in the previous problem.
Two charged particles with charge 2.0 × 10−8 C each are joined by an insulating string of length 1 m and the system is kept on a smooth horizontal table. Find the tension in the string.
Two identical balls, each with a charge of 2.00 × 10−7 C and a mass of 100 g, are suspended from a common point by two insulating strings, each 50 cm long. The balls are held at a separation 5.0 cm apart and then released. Find.
(a) the electric force on one of the charged balls
(b) the components of the resultant force on it along and perpendicular to the string
(c) the tension in the string
(d) the acceleration of one of the balls. Answers are to be obtained only for the instant just after the release.
Two small spheres, each with a mass of 20 g, are suspended from a common point by two insulating strings of length 40 cm each. The spheres are identically charged and the separation between the balls at equilibrium is found to be 4 cm. Find the charge on each sphere.
Two identically-charged particles are fastened to the two ends of a spring of spring constant 100 N m−1 and natural length 10 cm. The system rests on a smooth horizontal table. If the charge on each particle is 2.0 × 10−8 C, find the extension in the length of the spring. Assume that the extension is small as compared to the natural length. Justify this assumption after you solve the problem.
Two particles A and B, each carrying a charge Q, are held fixed with a separation dbetween them. A particle C of mass m and charge q is kept at the middle point of the line AB. Under what conditions will the particle C execute simple harmonic motion if it is released after such a small displacement? Find the time period of the oscillations if these conditions are satisfied.
How much work has to be done in assembling three charged particles at the vertices of an equilateral triangle, as shown in the figure?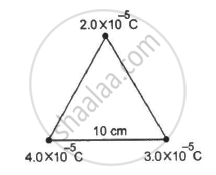
A total charge Q is broken in two parts Q1 and Q2 and they are placed at a distance R from each other. The maximum force of repulsion between them will occur, when ____________.
The unit of charge is ______.
A charge Q is divided into two parts of q and Q – q. If the coulomb repulsion between them when they are separated is to be maximum, the ratio of Q/q should be ______.
