Advertisements
Advertisements
Question
A solid is in the shape of a cone surmounted on a hemisphere, the radius of each of them being 3.5 cm and the total height of the solid is 9.5 cm. Find the volume of the solid.
Solution

We have,
Radius of cone = radius of hemisphere =r = 3.5 cm or AD = BD = CI
Total height of the solid, OC = 9.5 cm
`rArr OD + CD = 9.5`
`rArr OD + 3.5 = 9.5`
`rArr OD = 6 cm`
⇒ Height of cone, h = 6 cm
Now,
Volume of solid = Volume of cone + Volume of hemisphere
`= 1/3pir^2h + 2/3pir^3`
`=1/3 pir^2 (h + 2r)`
`=1/3xx22/7xx3.5xx3.5xx(6+2xx3.5)`
`= 1/3xx 22/7xx3.5xx3.5xx(6+7)`
`= 1/3xx22/7xx3.5xx3.5xx13`
`= 500.5/3`
≈ 166.83 cm3
So, the volume of the solid is 166.83 cm3.
RELATED QUESTIONS
The diameters of the internal and external surfaces of a hollow spherical shell are 6 cm and 10 cm respectively. If it is melted and recast and recast into a solid cylinder of diameter 14 cm, find the height of the cylinder.
A hollow sphere of internal and external diameter 4cm and 8cm is melted into a cone of base diameter 8cm. Calculate height of cone?
A reservoir in the form of the frustum of a right circular cone contains 44 × 107 litres of water which fills it completely. The radii of the bottom and top of the reservoir are 50 metres and 100 metres respectively. Find the depth of water and the lateral surface area of the reservoir. (Take: π = 22/7)
Marbles of diameter 1.4 cm are dropped into a cylindrical beaker of diameter 7 cm, containing some water. Find the number of marbles that should be dropped into the beaker so that the water level rises by 5.6 cm.
A surahi is a combination of
A hemispherical bowl of internal diameter 30 cm is full of a liquid. This liquid is poured into cylindrical bottles of diameter 5 cm and height 6 cm each. How many bottles are required?
The sum of the inner and the outer curved surfaces of a hollow metallic cylinder is 1056 cm2 and the volume of material in it is 1056 cm3. Find its internal and external radii. Given that the height of the cylinder is 21 cm.
Water in a canal, 6 m wide and 1.5 m deep, is flowing with a speed of 10 km/hour. How much area will it irrigate in 30 minutes; if 8 cm standing water is needed?
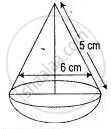
The given figure represents a hemisphere surmounted by a conical block of wood. The diameter of their bases is 6 cm each and the slant height of the cone is 5 cm. Calculate:
(i) the height of the cone.
(ii) the vol. of the solid.
Three metallic solid cubes whose edges are 3 cm, 4 cm and 5 cm are melted and formed into a single cube. Find the edge of the cube so formed.
