Advertisements
Advertisements
Question
ABCD is a rhombus and its diagonals intersect at O.
(i) Is ∆BOC ≅ ∆DOC? State the congruence condition used?
(ii) Also state, if ∠BCO = ∠DCO.
Advertisements
Solution
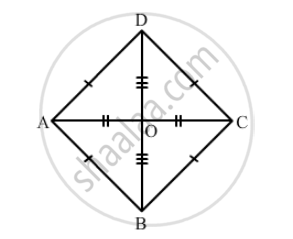
(i) Yes
\[\text{ In } ∆ BCO \text{ and } ∆ DCO: \]
\[OC = OC (\text{ common })\]
\[BC = DC (\text{ all sides of a rhombus are equal })\]
\[BO = OD (\text{ diagonals of a rhomus bisect each other })\]
\[\text{ By SSS congruence }: \]
\[ ∆ BCO \cong ∆ DCO\]
Yes
By c.p.c.t:
\[\angle BCO = \angle DCO\]
RELATED QUESTIONS
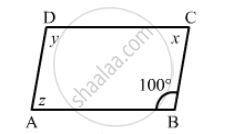
The following figure is parallelogram. Find the degree values of the unknown x, y, z.
In the adjacent figure HOPE is a parallelogram. Find the angle measures x,y and z. State the geometrical truths you use to find them.
Two opposite angles of a parallelogram are (3x − 2)° and (50 − x)°. Find the measure of each angle of the parallelogram.
Which of the following statement is true for a rhombus?
It has only two pairs of equal sides.
Fill in the blank, inthe following, so as to make the statement true:
A square is a rhombus in which .....
Fill in the blank, in each of the following, so as to make the statement true:
If the diagonals of a parallelogram bisect each other at right angles, then it is a ......
Draw a rhombus, having each side of length 3.5 cm and one of the angles as 40°.
Diagonals of a rhombus are 20 cm and 21 cm respectively, then find the side of rhombus and its perimeter.
If opposite angles of a rhombus are (2x)° and (3x - 40)° then value of x is ______.
In the figure, BEST is a rhombus, Then the value of y – x is ______.
