Advertisements
Advertisements
Question
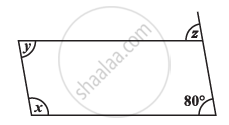
Consider the given parallelograms. Find the values of the unknowns x, y, z.

Solution
x = 90° (Vertically opposite angles)
x + y + 30° = 180° (Angle sum property of triangles)
90° + 30° + y = 180°
120° + y = 180°
y = 180° − 120° = 60° ...[Since alternate angles are equal in a parallelogram]
y = z = 60°
Thus x = 90°, y = 60° and z = 60°
APPEARS IN
RELATED QUESTIONS
Consider the given parallelograms. Find the values of the unknowns x, y, z.
In the given figure, if points P, Q, R, S are on the sides of parallelogram such that AP = BQ = CR = DS then prove that `square`PQRS is a parallelogram.

Prove that the diagonals of a parallelogram bisect each other.
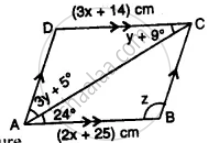
Use the information given in the alongside diagram to find the value of x, y, and z.
If a triangle and a parallelogram lie on the same base and between the same parallels, then prove that the area of the triangle is equal to half of the area of parallelogram
Iron rods a, b, c, d, e, and f are making a design in a bridge as shown in the figure. If a || b, c || d, e || f, find the marked angles between d and e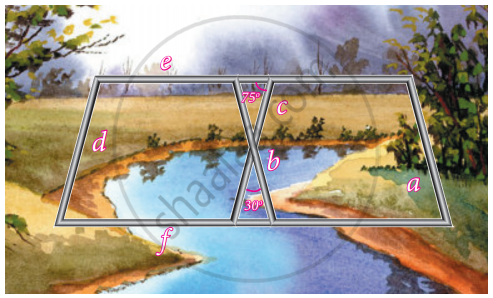
In parallelogram LOST, SN ⊥ OL and SM ⊥ LT. Find ∠STM, ∠SON and ∠NSM.

Two angles of a quadrilateral are each of measure 75° and the other two angles are equal. What is the measure of these two angles? Name the possible figures so formed.
The angle between the two altitudes of a parallelogram through the vertex of an obtuse angle of the parallelogram is 45°. Find the angles of the parallelogram.
In parallelogram ABCD, the angle bisector of ∠A bisects BC. Will angle bisector of B also bisect AD? Give reason.
