Advertisements
Advertisements
Question
Construct a tangent to a circle of radius 4 cm from a point on the concentric circle of radius 6 cm and measure its length. Also verify the measurement by actual calculation.
Solution 1
In order to do the desired construction,

we follow the following steps:
Step I: Take a point O on the plane of the paper and draw a circle of radius OA = 4 cm. Also, draw a concentric circle of radius OB = 6 cm.
Step II: Find the mid-point C of OB and draw a circle of radius OC = BC. Suppose this circle intersects the circle of radius 4 cm at P and Q.
Step III: Join BP and BQ to get the desired tangents from a point B on the circle of radius 6 cm.
By actual measurement, we find the
BP = BQ = 4.5 cm
Justification: In ∆BPO, we have
OB = 6 cm and OP = 4 cm
`OB^2 = BP^2 + OP^2 ` [Using Pythagoras theorem]
`\Rightarrow BP=\sqrt{OB^{2}-OP^{2}`
`\Rightarrow \sqrt{36-16}=\sqrt{20}=4.47 ~= 4.5 `
Similarly, BQ = 4.47cm ≈ 4.5 cm
Solution 2
Tangents on the given circle can be drawn as follows.
Step 1
Draw a circle of 4 cm radius with centre as O on the given plane.
Step 2
Draw a circle of 6 cm radius taking O as its centre. Locate a point P on this circle and join OP.
Step 3
Bisect OP. Let M be the mid-point of PO.
Step 4
Taking M as its centre and MO as its radius, draw a circle. Let it intersect the given circle at the points Q and R.
Step 5
Join PQ and PR. PQ and PR are the required tangents.
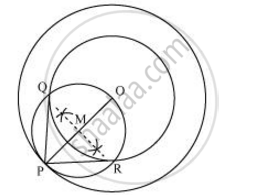
It can be observed that PQ and PR are of length 4.47 cm each.
In ΔPQO,
Since PQ is a tangent,
∠PQO = 90°
PO = 6 cm
QO = 4 cm
Applying Pythagoras theorem in ΔPQO, we obtain
PQ2 + QO2 = PQ2
PQ2 + (4)2 = (6)2
PQ2 + 16 = 36
PQ2 = 36 − 16
PQ2 = 20
PQ = `2sqrt5`
PQ = 4.47 cm
Justification
The construction can be justified by proving that PQ and PR are the tangents to the circle (whose centre is O and radius is 4 cm). For this, let us join OQ and OR.

∠PQO is an angle in the semi-circle. We know that angle in a semi-circle is a right angle.
∴ ∠PQO = 90°
⇒ OQ ⊥ PQ
Since OQ is the radius of the circle, PQ has to be a tangent of the circle. Similarly, PR is a tangent of the circle.
APPEARS IN
RELATED QUESTIONS
Draw a line AB = 5 cm. Mark a point C on AB such that AC = 3 cm. Using a ruler and a compass only, construct :
- A circle of radius 2.5 cm, passing through A and C.
- Construct two tangents to the circle from the external point B. Measure and record the length of the tangents.
Draw a line segment AB of length 8 cm. Taking A as centre , draw a circle of radius 4 cm and taking B as centre , draw another circle of radius 3 cm. Construct tangents to each circle form the centre of the other circle.
Draw a pair of tangents to a circle of radius 3 cm, which are inclined to each other at an angle of 60°.
In Figure 2, PQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q intersect at a point T. Find the length TP.

Draw a circle at a radius of 4 cm. Take a point on it. Without using the centre at the circle, draw a tangent to the circle at point P.
Draw two lines AB, AC so that ∠ BAC = 40°:
(i) Construct the locus of the center of a circle that touches AB and has a radius of 3.5 cm.
(ii) Construct a circle of radius 35 cm, that touches both AB and AC, and whose center lies within the ∠ BAC.
Draw a circle of radius 3 cm. Construct a square about the circle.
A pair of tangents can be constructed from a point P to a circle of radius 3.5 cm situated at a distance of 3 cm from the centre.
Construct a pair of tangents to a circle of radius 4 cm from a point P lying outside the circle at a distance of 6 cm from the centre.
Using ruler and compass construct a triangle ABC in which AB = 6 cm, ∠BAC = 120° and AC = 5 cm. Construct a circle passing through A, B and C. Measure and write down the radius of the circle.
