Advertisements
Advertisements
Question
Define the term "cut off frequency" in photoelectric emission. The threshod frequency of a metal is f. When the light of frequency 2f is incident on the metal plate, the maximum velocity of photo-electrons is v1. When the frequency of the incident radiation is increased to 5f, the maximum velocity of phto-electrons is v2. Find the ratio v1 : v2.
Solution
That particular frequency of incident radiation for which the minimum negative potential V0 given to a plate for photelectric current becomes zero is called the cut-off frequency.
Given that threshold frequency of metal is f. For light of frequency 2f, using Einstein's equation for photoelectric effect, we can write
\[h\left( 2f - f \right) = \frac{1}{2}m {v_1}^2\] .......... (1)
Similarly, for light having frequency 5f, we have
\[\frac{f}{4f} = \frac{{v_1}^2}{{v_2}^2} \Rightarrow \frac{v_1}{v_2} = \sqrt{\frac{1}{4}}\]
\[ \Rightarrow \frac{v_1}{v_2} = \frac{1}{2}\]
APPEARS IN
RELATED QUESTIONS
The threshold frequency for a certain metal is 3.3 × 1014 Hz. If light of frequency 8.2 × 1014 Hz is incident on the metal, predict the cutoff voltage for the photoelectric emission.
Is the formula you employ in (a) valid for calculating radius of the path of a 20 MeV electron beam? If not, in what way is it modified?
What is so special about the combination e/m? Why do we not simply talk of e and m separately?
Two neutral particles are kept 1 m apart. Suppose by some mechanism some charge is transferred from one particle to the other and the electric potential energy lost is completely converted into a photon. Calculate the longest and the next smaller wavelength of the photon possible.
(Use h = 6.63 × 10-34J-s = 4.14 × 10-15 eV-s, c = 3 × 108 m/s and me = 9.1 × 10-31kg)
A light beam of wavelength 400 nm is incident on a metal plate of work function 2.2 eV. (a) A particular electron absorbs a photon and makes two collisions before coming out of the metal. Assuming that 10% of the extra energy is lost to the metal in each collision, find the kinetic energy of this electron as it comes out of the metal. (b) Under the same assumptions, find the maximum number of collisions the electron can suffer before it becomes unable to come out of the metal.
Plot a graph to show the variation of stopping potential with frequency of incident radiation in relation to photoelectric effect.
The stopping potential in an experiment on photoelectric effect is 1.5V. What is the maximum kinetic energy of the photoelectrons emitted? Calculate in Joules.
For a given frequency of light and a positive plate potential in the set up below, If the intensity of light is increased then ______.
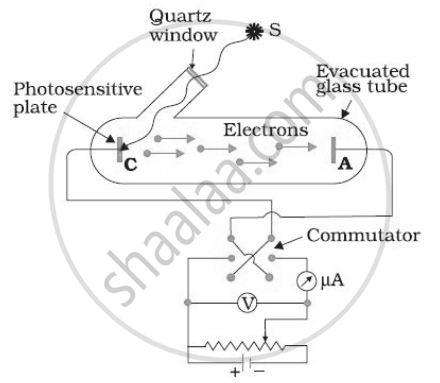
In various experiments on photo electricity, the stopping potential for a given frequency of the incident radiation is ______.
In photoelectric effect, the photoelectric current
