Advertisements
Advertisements
Question
Divide a line segment of length 14 cm internally in the ratio 2 : 5. Also, justify your construction.
Solution
Given that
Determine a point which divides a line segment of length 14cm internally in the ratio of 2:5.
We follow the following steps to construct the given
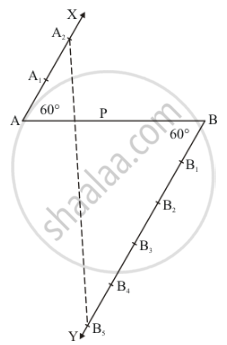
Step of construction
Step: I-First of all we draw a line segment AB = 14cm.
Step: II- We draw a ray AX making an acute angle ∠BAX = 60° with AB.
Step: III- Draw a ray BY parallel to AX by making an acute angle ∠ABY = ∠BAX.
Step IV- Mark of two points A1, A2 on AX and three points B1, B2, B3, B4, B5 on BY in such a way that AAA1 = A1A2 = B1B2 = B2B3 = B3B4 = B4B5.
Step: V- Joins A2B3 and this line intersects AB at a point P.
Thus, P is the point dividing AB internally in the ratio of 2:5
Justification:-
In ΔAA2P and ΔBB5P we have
∠A2AP = ∠PBB5 [∠ABY = ∠BAX]
And ∠APA2 = ∠BPB5 [Vertically opposite angle]
So, AA similarity criterion, we have
ΔAA2P ≈ ΔBB5P
`(A A_2)/(BB_5)=(AP)(BP)`
`(AP)/(BP)=2/5`
APPEARS IN
RELATED QUESTIONS
Determine a point which divides a line segment of length 12 cm internally in the ratio 2 : 3 Also, justify your construction.
Construct a triangle similar to a given ΔABC such that each of its sides is (5/7)th of the corresponding sides of Δ ABC. It is given that AB = 5 cm, BC = 7 cm and ∠ABC = 50°.
Draw a right triangle in which the sides (other than hypotenuse) are of lengths 5 cm and 4 cm. Then construct another triangle whose sides are 5/3th times the corresponding sides of the given triangle.
Construct a triangle similar to a given ΔXYZ with its sides equal to (3/4)th of the corresponding sides of ΔXYZ. Write the steps of construction.
Draw a line segment AB of length 7 cm. Using ruler and compasses, find a point P on AB such that `(AP)/(AB) = 3/5 `.
Construct an equilateral ∆ABC with side 5 cm. ∆ABC ~ ∆LMN, ratio the corresponding sides of triangle is 6 : 7, then construct ΔLMN and ΔABC
ΔABC ~ ΔPBR, BC = 8 cm, AC = 10 cm , ∠B = 90°, `"BC"/"BR" = 5/4` then construct ∆ABC and ΔPBR
To divide a line segment AB in the ratio 4 : 7, a ray AX is drawn first such that ∠BAX is an acute angle and then points A1, A2, A3, .... are located at equal distances on the ray AX and the point B is joined to ______.
If I ask you to construct ΔPQR ~ ΔABC exactly (when we say exactly, we mean the exact relative positions of the triangles) as given in the figure, (Assuming I give you the dimensions of ΔABC and the Scale Factor for ΔPQR) what additional information would you ask for?
Draw a parallelogram ABCD in which BC = 5 cm, AB = 3 cm and ∠ABC = 60°, divide it into triangles BCD and ABD by the diagonal BD. Construct the triangle BD' C' similar to ∆BDC with scale factor `4/3`. Draw the line segment D'A' parallel to DA where A' lies on extended side BA. Is A'BC'D' a parallelogram?
