Advertisements
Advertisements
Question
Draw a circle of radius 4 cm. Take a point P outside the circle without using the center at the circle. Draw two tangents to the circle from point P.
Solution
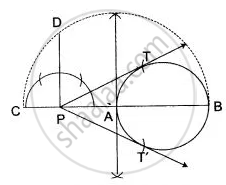
Steps of construction:
(i) Draw a circle of radius 4 cm.
(ii) Take a point P outside the circle and draw a secant PAB, intersecting the circle at A and B.
(iii) Produce AP to C such that AP = CP.
(iv) Draw a semicircle with CB as diameter.
(v) Draw PD and CB intersecting the semi-circle at D.
(vi) With P as centre and PD as a radius draw arcs to intersect the given circle at T and T'.
(vii) Join PT and PT'. Then PT and PT' are the required tangents.
APPEARS IN
RELATED QUESTIONS
Draw a right triangle ABC in which AB = 6 cm, BC = 8 cm and ∠B = 90°. Draw BD perpendicular from B on AC and draw a circle passing through the points B, C and D. Construct tangents from A to this circle.
Draw a circle with the help of a bangle. Take a point outside the circle. Construct the pair of tangents from this point to the circles. Give the justification of the construction.
Draw a circle of radius 3.5 cm. Take two points A and B on one of its extended diameter, each at a distance of 5 cm from its center. Draw tangents to the circle from each of these points A and B.
Draw a circle at a radius of 3 cm. Take a point at 5.5 cm from the center at the circle. From point P, draw two tangents to the circle.
To draw a pair of tangents to a circle which are inclined to each other at an angle of 60°, it is required to draw tangents at end points of those two radii of the circle, the angle between them should be ______.
A circle of radius r has a center O. What is first step to construct a tangent from a generic point P which is at a distance r from O?
A pair of tangents can be constructed from a point P to a circle of radius 3.5 cm situated at a distance of 3 cm from the centre.
A pair of tangents can be constructed to a circle inclined at an angle of 170°.
Construct a pair of tangents to a circle of radius 3 cm which are inclined to each other at an angle of 60°.
Using ruler and compass construct a triangle ABC in which AB = 6 cm, ∠BAC = 120° and AC = 5 cm. Construct a circle passing through A, B and C. Measure and write down the radius of the circle.
