Advertisements
Advertisements
Question
Evaluate `int int xy(x-1)dx dy` over the region bounded by ๐๐ = ๐,๐= ๐,๐ =๐ and ๐ = ๐
Solution
Let I = `int int xy(x-1)dx dy`
Rectangular hyperbola : ๐๐=๐ Lines : ๐=๐ ,๐=๐ ,๐=๐
Intersection of line ๐ = ๐ and ๐๐ = ๐ is (1,4).
Intersection of line ๐ = ๐ and ๐๐ = ๐ is (4,1)
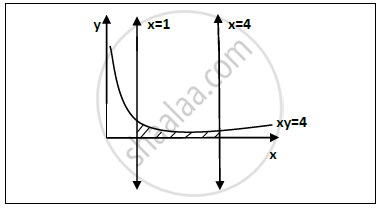
`therefore 0<=y<=x/4`
`1<=x<=4`
`therefore "I" =int_1^4 int_0^(x/4)(x^2y-xy)dy dx`
`=int_1^4[y^2/2x^2-(y^2x)/2]_0^(x/4)dx`
`=int_1^4(8-8/x)dx`
`=[8x-8logx]_1^4`
`therefore "I"=8(3-2log2)`
APPEARS IN
RELATED QUESTIONS
Find the area inside the circle r=a sin๐ฝ and outside the cardioide r=a(1+cos๐ฝ )
Evaluate `int int(2xy^5)/sqrt(x^2y^2-y^4+1)dxdy`, where R is triangle whose vertices are (0,0),(1,1),(0,1).
Use polar co ordinates to evaluate `int int (x^2+y^2)^2/(x^2y^2)` ๐ ๐ ๐ ๐ over yhe area Common to circle `x^2+y^2=ax "and" x^2+y^2=by, a>b>0`
Find by double integration the area bounded by the parabola ๐๐=๐๐ And ๐=๐๐−๐
