Advertisements
Advertisements
Question
Figure shows Δ KLM , P an T on KL and KM respectively such that∠ KLM =∠ KTP.
If `"KL"/"KT" = 9/5` , find `("Ar" triangle "KLM")/("Ar" triangle "KTP")`.

Solution
Given : `"KL"/"KT"= 9/5`
To find : `("Ar" triangle "KLM")/("Ar" triangle "KTP")`
Sol : In Δ KLM and Δ KTP
∠ KLM =∠ KTP ....(Given)
∠ LKM = ∠ TKP ....(common)
Δ KLM and Δ KTP ......(AA corollary)
`therefore ("Ar" triangle "KLM")/("Ar" triangle "KTP") = ("KL"/"KT")^2 = (9/5)^2 = 81/25`
i.e., 81 : 25
[The ration of areas of two similar triangle is equal to the ratio of square of their corresponding sides.]
APPEARS IN
RELATED QUESTIONS
Using Basic proportionality theorem, prove that a line drawn through the mid-points of one side of a triangle parallel to another side bisects the third side. (Recall that you have proved it in Class IX).
The corresponding altitudes of two similar triangles are 6cm and 9cm respectively. Find the ratio of their areas.
In the given figure, X is any point in the interior of triangle. Point X is joined to vertices of triangle. Seg PQ || seg DE, seg QR || seg EF. Fill in the blanks to prove that, seg PR || seg DF.

Proof : In ΔXDE, PQ || DE ...`square`
∴ `"XP"/square = square/"QE"` ...(I) (Basic proportionality theorem)
In ΔXEF, QR || EF ...`square`
∴ `square/square = square/square ..."(II)" square`
∴ `square/square = square/square` ...from (I) and (II)
∴ seg PR || seg DF ...(converse of basic proportionality theorem)
In the following figure, point D divides AB in the ratio 3 : 5. Find : `(AE)/(AC)`

In ΔABC, point D divides AB in the ratio 5:7, Find: `"AE"/"AC"`
ΔABC is enlarged, with a scale factor 5. Find: BC, f B'C' = 16cm
D is the mid point of side BC and AE ⊥ BC. If BC = a, AC = b, AB = c, ED = x, AD = p and AE = h, prove that c2 = `"p"^2 - "a"x + "a"^2/4`
In the figure, if ∠FEG ≡ ∠1 then, prove that DG2 = DE.DF
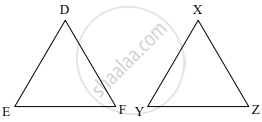
In ΔDEF and ΔXYZ, `"DE"/"XY" = "FE"/"YZ"` and ∠E ≅ ∠Y. _______ test gives similarity between ΔDEF and ΔXYZ.
In figure, if AD = 6 cm, DB = 9 cm, AE = 8 cm and EC = 12 cm and ∠ADE = 48°. Find ∠ABC.

