Advertisements
Advertisements
Question
Find the area of the region bounded by the parabola y2 = 16x and the line x = 4
Solution
Given equation of the curve is y2 = 16x
y2 =
∴ y = `+- 4sqrt(x)`
∴ y = `4 sqrt(x)` ......[∵ In first quadrant, y > 0]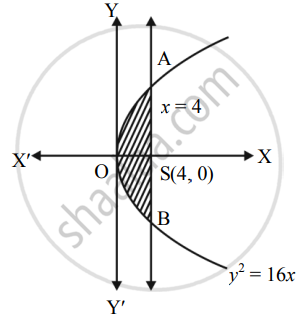
Required area = Area of the region OBSAO
= 2 .......(Area of the region OSAO)
= `2int_0^4 y "d"x`
= `2 int_0^4 4sqrt(x) "d"x`
= `8[(x^(3/2))/(3/2)]_0^4`
= `16/3[(4)^(3/2) - 0]`
= `16/3(8)`
= `128/3` sq.units
APPEARS IN
RELATED QUESTIONS
Find the area of the region bounded by the following curves, X-axis and the given lines : x = 0, x = 5, y = 0, y = 4
Find the area of the region bounded by the following curves, X-axis and the given lines : y2 = x, x = 0, x = 4
Find the area of the region bounded by the following curves, X-axis and the given lines: y2 = 16x, x = 0, x = 4
Find the area of the region included between y2 = 2x and y = 2x.
Choose the correct option from the given alternatives :
The area bounded by the regional 1 ≤ x ≤ 5 and 2 ≤ y ≤ 5 is given by ______.
Choose the correct option from the given alternatives :
The area bounded by the curve y = x3, the X-axis and the lines x = – 2 and x = 1 is
The area of the region bounded by y = cos x, Y-axis and the lines x = 0, x = 2π is ______.
Choose the correct option from the given alternatives :
The area of the circle x2 + y2 = 25 in first quadrant is
Choose the correct option from the given alternatives :
The area enclosed between the curve y = cos 3x, 0 ≤ x ≤ `pi/(6)` and the X-axis is
Choose the correct option from the given alternatives :
The area bounded by y = `sqrt(x)` and the x = 2y + 3, X-axis in first quadrant is
Choose the correct option from the given alternatives :
The area bounded by the ellipse `x^2/a^2 y^2/b^2` = 1 and the line `x/a + y/b` = 1 is
The area bounded by the curve y = tan x, X-axis and the line x = `pi/(4)` is ______.
Choose the correct option from the given alternatives :
The area of the region bounded by x2 = 16y, y = 1, y = 4 and x = 0 in the first quadrant, is
Solve the following :
Find the area of the region bounded by the following curve, the X-axis and the given lines : y = sin x, x = 0, x = π
Solve the following :
Find the area of the region lying between the parabolas : 4y2 = 9x and 3x2 = 16y
Solve the following :
Find the area of the region lying between the parabolas : y2 = x and x2 = y.
Solve the following :
Find the area of the region bounded by the curve y = 4x2, Y-axis and the lines y = 1, y = 4.
The area of the region bounded by the ellipse x2/64 + y2/100 = 1, is ______ sq.units
The area bounded by the parabola y2 = x along the X-axis and the lines x = 0, x = 2 is ______ sq.units
Find the area bounded by the curve y = sin x, the lines x = 0 and x = `pi/2`
Find the area of the region bounded by the curve y = x2, the X−axis and the given lines x = 0, x = 3
Find the area of the region bounded by the curve y2 = 8x, the X−axis and the given lines x = 1, x = 3, y ≥ 0
Find the area of the region bounded by the parabola x2 = 4y and The X-axis and the line x = 1, x = 4
Find the area of the region bounded by the curves x2 = 8y, y = 2, y = 4 and the Y-axis, lying in the first quadrant
Find the area of the region bounded by the curve y = sin x, the X−axis and the given lines x = − π, x = π
Find the area of the region bounded by the curves y2 = 4ax and x2 = 4ay
Find the area of the region bounded by the curve (y − 1)2 = 4(x + 1) and the line y = (x − 1)
The area bounded by the curve y = x3, the X-axis and the Lines x = –2 and x = 1 is ______.
Find the area of the region lying in the first quadrant and bounded by y = 4x2, x = 0, y = 2 and y = 4.
Find the area of the region bounded by the curve y = x2, and the lines x = 1, x = 2, and y = 0.
