Advertisements
Advertisements
Question
Find the area of the trapezium ABCD in which AB || DC, AB = 18 cm, ∠B = ∠C = 90°, CD = 12 cm and AD = 10 cm.
Solution
In trapezium ABCD,
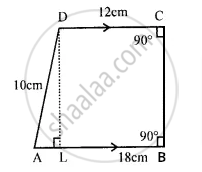
AB || DC, AB = 18 cm
∠B = ∠C = 90°, CD = 12 cm and AD = 10 cm.
Area of trapezium ABCD
Draw DL ⊥ AB
∴ AL = 18 - 12 = 6 cm
DL = BC
DL = `sqrt("AD"^2 - "AL"^2)`
= `sqrt(10^2 - 6^2) = sqrt(100 - 36)`
= `sqrt(64) = 8 "cm"`
Now area of trapezium = `1/2 ("AB + CD") xx "DL"`
= `1/2(18 + 12) xx 8 "cm"^2`
= `1/2 xx 30 xx 8 = 120 "cm"^2`
APPEARS IN
RELATED QUESTIONS
Find the area, in square metres, of the trapezium whose bases and altitude is as under:
bases = 12 dm and 20 dm, altitude = 10 dm
Find the area, in square metres, of the trapezium whose bases and altitude is as under:
bases = 28 cm and 3 dm, altitude = 25 cm
Find the area of a trapezium whose parallel sides of lengths 10 cm and 15 cm are at a distance of 6 cm from each other. Calculate this area as the difference of the area of a rectangle and the sum of the areas of two triangles.
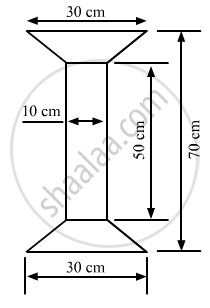
Find the area of Fig. as the sum of the areas of two trapezium and a rectangle.
The cross-section of a canal is a trapezium in shape. If the canal is 10 m wide at the top 6 m wide at the bottom and the area of cross-section is 72 m2 determine its depth.
If the area of a trapezium is 28 cm2 and one of its parallel sides is 6 cm, find the other parallel side if its altitude is 4 cm.
Find the area of the field shown in Fig. 20.39 by dividing it into a square, a rectangle and a trapezium.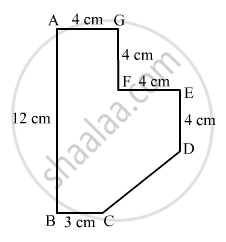
The area of a trapezium is 180 sq.cm and its height is 9 cm. If one of the parallel sides is longer than the other by 6 cm. Find the length of the parallel sides.
The area of the trapezium, if the parallel sides are measuring 8 cm and 10 cm and the height 5 cm is
In a trapezium if the sum of the parallel sides is 10 cm and the area is 140 sq.cm, then the height is
