Advertisements
Advertisements
Question
Find the equation of the lines passing through the point (1, 1) and the perpendicular from the origin makes an angle 60° with x-axis
Solution
The equation of the line in the normal form is x cos α + y sin α = p ......(1)
Given α = 60°
∴ cos 60° = `1/2`, sin 60° = `sqrt(3)/2`
(1) ⇒ `x * 1/2 +y * sqrt(3)/2` = p
`x + sqrt(3)` = 2p .......(2)
This line passes through the point (1, 1)
∴ `1 + sqrt(3)` = 2p
Substituting for p in equation (2)
The required equation is `x + sqrt(3)y = 1 + sqrt(3)`
APPEARS IN
RELATED QUESTIONS
Find the slope of the following line which passes through the points:
G(7, 1), H(−3, 1)
Find the slope of the line whose inclination is `pi/4`
Find the slope of the line which makes angle of 45° with the positive direction of the Y-axis measured anticlockwise
Find the value of k for which points P(k, −1), Q(2, 1) and R(4, 5) are collinear.
Find the acute angle between the X-axis and the line joining points A(3, −1) and B(4, −2).
If points A(h, 0), B(0, k) and C(a, b) lie on a line then show that `"a"/"h" + "b"/"k"` = 1
Answer the following question:
Find the value of k the point P(1, k) lies on the line passing through the points A(2, 2) and B(3, 3)
The normal boiling point of water is 100°C or 212°F and the freezing point of water is 0°C or 32°F. Find the linear relationship between C and F
The normal boiling point of water is 100°C or 212°F and the freezing point of water is 0°C or 32°F. Find the value of C for 98.6°F
The normal boiling point of water is 100°C or 212°F and the freezing point of water is 0°C or 32°F. Find the value of F for 38°C
An object was launched from a place P in constant speed to hit a target. At the 15th second, it was 1400 m from the target, and at the 18th second 800 m away. Find the distance between the place and the target
An object was launched from a place P in constant speed to hit a target. At the 15th second, it was 1400 m from the target, and at the 18th second 800 m away. Find time taken to hit the target
A 150 m long train is moving with constant velocity of 12.5 m/s. Find time taken to cross the bridge of length 850 m
In a shopping mall there is a hall of cuboid shape with dimension 800 × 800 × 720 units, which needs to be added the facility of an escalator in the path as shown by the dotted line in the figure. Find the slopes of the escalator at the turning points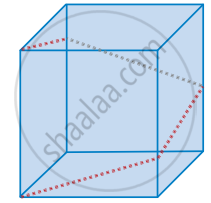
Choose the correct alternative:
The line (p + 2q)x + (p − 3q)y = p − q for different values of p and q passes through the point
If one of the lines given by kx2 + 2xy – 3y2 = 0 is perpendicular to the line 3x + 5y+ 1 = 0, then the value of k is ______.
Find the transformed equation of the straight line 2x – 3y + 5 = 0, when the origin is shifted to the point (3, –1) after translation of axes.
