Advertisements
Advertisements
Question
Find the length of the perpendicular of a triangle whose base is 5cm and the hypotenuse is 13cm. Also, find its area.
Solution
Base = 5cm, Hypotenuse = 13cm
By Pythagoras theorem,
(perpendicular)2 = (13cm)2 - (5cm)2
(perpendicular)2 = 169cm2 - 25cm2
(perpendicular)2 = 144cm2
(perpendicular)2 = (12cm)2
∴ Perpendicular = 12cm
Area of the triangle
= 13cm2 x (Base x Perpendicular)
= `(1)/(2) xx 5"cm" xx 12"cm"`
= 30cm2.
APPEARS IN
RELATED QUESTIONS
In a right triangle ABC, right-angled at B, BC = 12 cm and AB = 5 cm. The radius of the circle inscribed in the triangle (in cm) is
(A) 4
(B) 3
(C) 2
(D) 1
A man goes 10 m due east and then 24 m due north. Find the distance from the starting point
In the given figure, ∆ABC is an equilateral triangle of side 3 units. Find the coordinates of the other two vertices ?

Identify, with reason, if the following is a Pythagorean triplet.
(11, 60, 61)
In right angle ΔABC, if ∠B = 90°, AB = 6, BC = 8, then find AC.
Find the value of (sin2 33 + sin2 57°)
In Fig. 3, ∠ACB = 90° and CD ⊥ AB, prove that CD2 = BD x AD.

In the given figure, BL and CM are medians of a ∆ABC right-angled at A. Prove that 4 (BL2 + CM2) = 5 BC2.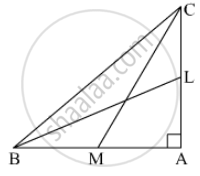
In triangle PQR, angle Q = 90°, find: PR, if PQ = 8 cm and QR = 6 cm
In the given figure, angle ACB = 90° = angle ACD. If AB = 10 m, BC = 6 cm and AD = 17 cm, find :
(i) AC
(ii) CD

In the given figure, angle ADB = 90°, AC = AB = 26 cm and BD = DC. If the length of AD = 24 cm; find the length of BC.

Calculate the area of a right-angled triangle whose hypotenuse is 65cm and one side is 16cm.
PQR is an isosceles triangle with PQ = PR = 10 cm and QR = 12 cm. Find the length of the perpendicular from P to QR.
If in a ΔPQR, PR2 = PQ2 + QR2, then the right angle of ∆PQR is at the vertex ________
An isosceles triangle has equal sides each 13 cm and a base 24 cm in length. Find its height
The hypotenuse of a right angled triangle of sides 12 cm and 16 cm is __________
A flag pole 18 m high casts a shadow 9.6 m long. Find the distance of the top of the pole from the far end of the shadow.
In a triangle, sum of squares of two sides is equal to the square of the third side.
Jayanti takes shortest route to her home by walking diagonally across a rectangular park. The park measures 60 metres × 80 metres. How much shorter is the route across the park than the route around its edges?
