Advertisements
Advertisements
Question
Find the value 'x', if: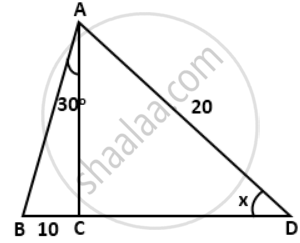
Solution

In right ΔACB,
tan30° = `"BC"/"AC"`
⇒ `(1)/sqrt(3) = (10)/"AC"`
⇒ AC = `10sqrt(3)"cm"`
Now,
In right ΔACD,
sin x = `"AC"/"AD"`
⇒ sin x = `(10sqrt(3))/(20)`
⇒ sin x = `sqrt(3)/(2)`
⇒ sin x = sin60°
⇒ x = 60°.
APPEARS IN
RELATED QUESTIONS
Solve the following equation for A, if sec 2A = 2
Solve the following equations for A, if `sqrt3` tan A = 1
Solve the following equation for A, if tan 3 A = 1
Solve for x : tan2 (x - 5°) = 3
Evaluate the following: `((sin3θ - 2sin4θ))/((cos3θ - 2cos4θ))` when 2θ = 30°
If θ < 90°, find the value of: sin2θ + cos2θ
If `sqrt(3)`sec 2θ = 2 and θ< 90°, find the value of θ
In the given figure, PQ = 6 cm, RQ = x cm and RP = 10 cm, find
a. cosθ
b. sin2θ- cos2θ
c. Use tanθ to find the value of RQ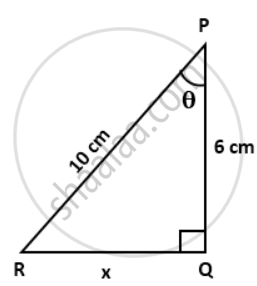
Evaluate the following: `(sin25° cos43°)/(sin47° cos 65°)`
Express each of the following in terms of trigonometric ratios of angles between 0° and 45°: sin53° + sec66° - sin50°
