Advertisements
Advertisements
Question
For a glass prism `(µ = sqrt(3))` the angle of minimum deviation is equal to the angle of the prism. Find the angle of the prism.
Solution
The refractive index of prism angle A and angle of minimum deviation `δ_m` is given by
`mu = (sin[((A + δ_m))/2])/(sin(A/2))`
Here we are given, δm = A
Substituting the value, we have `mu = (sin A)/(sin A/2)`
⇒ `mu = (2sin A/2 cos A/2)/(sin A/2) = 2 cos A/2`
⇒ `mu = (sin A)/(sin A/2) = (2sin A/2 cos A/2)/(sin A/2) = 2 cos A/2`
For the given value of refractive index,
We have, `cos A/2 = sqrt(3)/2 ⇒ A/2` = 30°
or A = 60°
This is the required value of prism angle.
APPEARS IN
RELATED QUESTIONS
At what angle should a ray of light be incident on the face of a prism of refracting angle 60° so that it just suffers total internal reflection at the other face? The refractive index of the material of the prism is 1.524.
How does the angle of minimum deviation of a glass prism vary, if the incident violet light is replaced by red light? Give reason.
Find the angle of incidence at face AB so that the emergent ray grazes along the face AC.
Can you ever have a situation in which a light ray goes undeviated through a prism?
A prism is made of glass of unknown refractive index. A parallel beam of light is incident on the face of the prism. The angle of minimum deviation is measured to be 40°. What is the refractive index of the material of the prism? The refracting angle of the prism is 60°. If the prism is placed in water (refractive index 1.33), predict the new angle of minimum deviation of a parallel beam of light.
The refractive index of a prism whose angle A = 60° is `sqrt2`. Then the angle of minimum deviation δm will be ______.
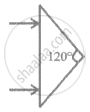
An isosceles prism of angle 120° has a refractive index 1.44. Two parallel monochromatic rays enter the prism parallel to each other in air as shown. The rays emerge from the opposite faces:
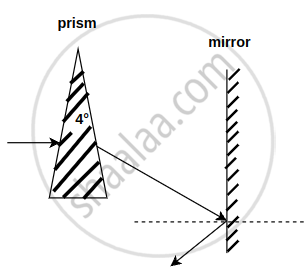
A horizontal ray of light passes through a prism of index 1.50 and apex angle 4° and then strikes a vertical mirror, as shown in the figure (a). Through what angle must the mirror be rotated if after reflection the ray is to be horizontal?
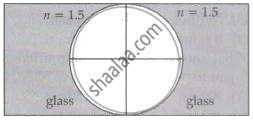
Two concave refracting surfaces of equal radii of curvature face each other in the air as shown in the figure. The point object O is placed midway between the centre and one of the poles. Then the separation between the images of O formed by each refracting surface is ______.
A ray of monochromatic light passes through an equilateral glass prism in such a way that the angle of incidence is equal to the angle of emergence and each of these angles is 3/4 times the angle of the prism. Determine the angle of deviation and the refractive index of the glass prism.
