Advertisements
Advertisements
Question
From the velocity – time graph given below, calculate Distance covered in the region ABCE.

Solution
Distance covered in region ABCE = ar(ΔABF) + area of trapezium BCEF
= `1/2xx"AF"xx"BF"+1/2("BF+CF")xx"GC"`
= `1/2xx12xx14+1/2(14+6)xx4`
= 6 × 14 + 10 × 4
= 84 + 40
= 124 m
APPEARS IN
RELATED QUESTIONS
What does the slope of velocity-time graph represent?
Multiple choice Question. Select the correct option.
In the velocity-time graph, the acceleration is

Draw velocity – time graph for the following situation:
When a body, is moving with uniform velocity.
Draw the following graph:
Speed versus time for a stepped motion
Draw the following graph:
Speed versus time for a uniformly retarded motion.
Draw distance-time graph to show:
Uniform velocity
Figure shows the distance-time graph of three students A, B and C. On the basis of the graph, answer the following :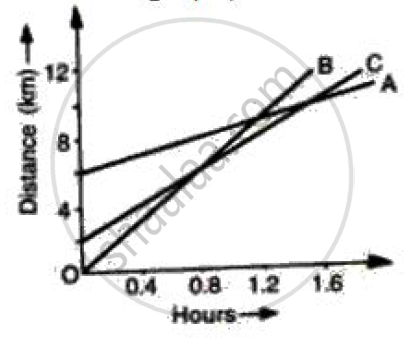
Which of the three is traveling the fastest?
Figure shows the distance-time graph of three students A, B and C. On the basis of the graph, answer the following :
How far did B travel between the time he passed C and A?
The area under velocity – time graph represents the ______
An object is moving in a positive direction with positive acceleration. The velocity-time graph with constant acceleration which represents the above situation is:
