Advertisements
Advertisements
Question
If (-3, 2), (1, -2) and (5, 6) are the midpoints of the sides of a triangle, find the coordinates of the vertices of the triangle.
Solution

let A(x1, y1 ), B(x2, y2) and O(x3 , y3) be the coordinates of the vertices of Δ ABC.
D is the midpoint of AB <
D(-3,2) = D `(("x"_1 + "x"_2)/2 , ("y"_1 + "y"_2)/2)`
`("x"_1 + "x"_2)/2 = -3 , ("y"_1 + "y"_2)/2`
X1 + X2 = -6 - - - (1) Y1 + Y2 = 4 .......(2)
Similarly
X2 + X3 = 2 - - - (3) Y2 + Y3 = -4 ......(4)
X1 + X3 = 10 - - - ( 5) Y1 + Y3 = 12 .......(6)
Adding (1), (3) and (5)
2(x1 + x2 + x,) = 6
x1 + x2 + x3 = 3
-6 + x3 = 3
x3 = 9
From (3)
x2 + 9 = 2
x2 = 9
From (3)
X2 + 9 = 2
X2 = -7
From (5)
x1 +9=10
x1 = 1
Adding (2), ( 4) and (6)
2(y1 + Y2+y3)= 12
Y1+ Y2 + Y3 = 6
4 + y3 = 6
Y3 = 2
from(4)
y2 + 2 = -4
Y2 = -6
from(6)
Y1+2= 12
Y1 = 10
The coordinates of the vertices of Δ ABC are (9,2), (1, 10) and (-7,-6).
APPEARS IN
RELATED QUESTIONS
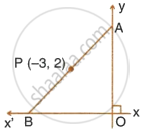
P(–3, 2) is the mid-point of line segment AB as shown in the given figure. Find the co-ordinates of points A and B.
Given a line ABCD in which AB = BC = CD, B = (0, 3) and C = (1, 8). Find the co-ordinates of A and D.
A(–1, 0), B(1, 3) and D(3, 5) are the vertices of a parallelogram ABCD. Find the co-ordinates of vertex C.
Calculate the co-ordinates of the centroid of the triangle ABC, if A = (7, –2), B = (0, 1) and C =(–1, 4).
(4, 2) and (-1, 5) are the adjacent vertices ofa parallelogram. (-3, 2) are the coordinates of the points of intersection of its diagonals. Find the coordinates of the other two vertices.
A triangle is formed by line segments joining the points (5, 1 ), (3, 4) and (1, 1). Find the coordinates of the centroid.
The coordinates of the end points of the diameter of a circle are (3, 1) and (7, 11). Find the coordinates of the centre of the circle.
The coordinates of the point C dividing the line segment joining the points P(2, 4) and Q(5, 7) internally in the ratio 2 : 1 is
Find the coordinates of the mid-point of the line segment with points A(– 2, 4) and B(–6, –6) on both ends.
Point P is the centre of the circle and AB is a diameter. Find the coordinates of points B if coordinates of point A and P are (2, – 3) and (– 2, 0) respectively.
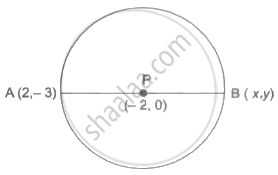
Given: A`square` and P`square`. Let B (x, y)
The centre of the circle is the midpoint of the diameter.
∴ Mid point formula,
`square = (square + x)/square`
⇒ `square = square` + x
⇒ x = `square - square`
⇒ x = – 6
and `square = (square + y)/2`
⇒ `square` + y = 0
⇒ y = 3
Hence coordinates of B is (– 6, 3).
