Advertisements
Advertisements
Question
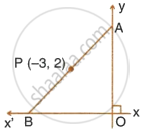
P(–3, 2) is the mid-point of line segment AB as shown in the given figure. Find the co-ordinates of points A and B.
Solution
Point A lies on y-axis, so let its co-ordinates be (0, y).
Point B lies on x-axis, so let its co-ordinates be (x, 0).
P(–3, 2) is the mid-point of line segment AB.
∴
Thus, the co-ordinates of points A and B are (0, 4) and (−6, 0) respectively.
APPEARS IN
RELATED QUESTIONS
Find the coordinates of point P if P divides the line segment joining the points A(–1, 7) and B(4, –3) in the ratio 2 : 3.
In the following example find the co-ordinate of point A which divides segment PQ in the ratio a : b.
P(–2, –5), Q(4, 3), a : b = 3 : 4
Find the midpoint of the line segment joining the following pair of point :
( -3, 5) and (9, -9)
Find the length of the median through the vertex A of triangle ABC whose vertices are A (7, -3), B(S, 3) and C(3, -1).
The centre of a circle is (a+2, a-1). Find the value of a, given that the circle passes through the points (2, -2) and (8, -2).
A , B and C are collinear points such that AB =
The midpoint of the line segment joining (2a, 4) and (-2, 2b) is (1, 2a+1). Find the value of a and b.
show that the points A(- 1, 2), B(2, 5) and C(- 5, – 2) are collinear.
The three vertices of a parallelogram taken in order are (-1, 0), (3, 1) and (2, 2) respectively. Find the coordinates of the fourth vertex.
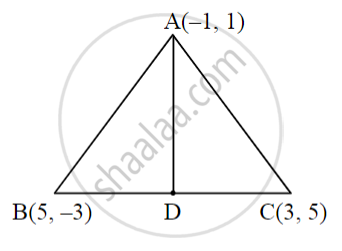
From the figure given alongside, find the length of the median AD of triangle ABC. Complete the activity.
Solution:
Here A(–1, 1), B(5, – 3), C(3, 5) and suppose D(x, y) are coordinates of point D.
Using midpoint formula,
x =
∴ x =
y =
∴ y =
Using distance formula,
∴ AD =
∴ AD =
∴ AD =
∴ The length of median AD =
ABC is a triangle whose vertices are A(1, –1), B(0, 4) and C(– 6, 4). D is the midpoint of BC. Find the coordinates of D.
