Advertisements
Advertisements
Question
If the points P, Q(x, 7), R, S(6, y) in this order divide the line segment joining A(2, p) and B(7, 10) in 5 equal parts, find x, y and p.
Solution
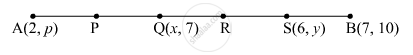
It is given that P, Q(x, 7), R, S(6, y) divides the line segment joining A(2, p) and B(7, 10) in 5 equal parts.
∴ AP = PQ = QR = RS = SB .....(1)
Now,
AP + PQ + QR + RS + SB = AB
⇒ SB + SB + SB + SB + SB = AB [From (1)]
⇒ 5SB = AB
⇒ SB = \[\frac{1}{5}\] AB .....(2)
Now,
AS = AP + PQ + QR + RS = \[\frac{1}{5}\] AB + \[\frac{1}{5}\] AB + \[\frac{1}{5}\] AB + \[\frac{1}{5}\] AB = \[\frac{4}{5}\] AB .....(3)
From (2) and (3), we get
AS : SB = \[\frac{4}{5}\] AB : \[\frac{1}{5}\] AB = 4 : 1
Similarly,
AQ : QB = 2 : 3
Using section formula, we get
Coordinates of Q =
\[ \Rightarrow 20 + 3p = 35\]
\[ \Rightarrow 3p = 15\]
\[ \Rightarrow p = 5\]
Thus, the values of x, y and p are 4, 9 and 5, respectively.
APPEARS IN
RELATED QUESTIONS
Prove that the points (3, 0), (6, 4) and (-1, 3) are the vertices of a right-angled isosceles triangle.
If A(–2, 1), B(a, 0), C(4, b) and D(1, 2) are the vertices of a parallelogram ABCD, find the values of a and b. Hence find the lengths of its sides
On which axis do the following points lie?
R(−4,0)
Show that the points (−3, 2), (−5,−5), (2, −3) and (4, 4) are the vertices of a rhombus. Find the area of this rhombus.
Prove that the points (3, 0), (4, 5), (-1, 4) and (-2, -1), taken in order, form a rhombus.
Also, find its area.
In the seating arrangement of desks in a classroom three students Rohini, Sandhya and Bina are seated at A(3, 1), B(6, 4), and C(8, 6). Do you think they are seated in a line?
Determine the ratio in which the straight line x - y - 2 = 0 divides the line segment
joining (3, -1) and (8, 9).
If the point P (2,2) is equidistant from the points A ( -2,K ) and B( -2K , -3) , find k. Also, find the length of AP.
The line segment joining A( 2,9) and B(6,3) is a diameter of a circle with center C. Find the coordinates of C
Find the area of quadrilateral PQRS whose vertices are P(-5, -3), Q(-4,-6),R(2, -3) and S(1,2).
Find the point on x-axis which is equidistant from points A(-1,0) and B(5,0)
If P is a point on x-axis such that its distance from the origin is 3 units, then the coordinates of a point Q on OY such that OP = OQ, are
If points A (5, p) B (1, 5), C (2, 1) and D (6, 2) form a square ABCD, then p =
The coordinates of the circumcentre of the triangle formed by the points O (0, 0), A (a, 0 and B (0, b) are
f the coordinates of one end of a diameter of a circle are (2, 3) and the coordinates of its centre are (−2, 5), then the coordinates of the other end of the diameter are
In Fig. 14.46, the area of ΔABC (in square units) is

If P(2, 4), Q(0, 3), R(3, 6) and S(5, y) are the vertices of a parallelogram PQRS, then the value of y is
Find the coordinates of the point whose ordinate is – 4 and which lies on y-axis.
If the points P(1, 2), Q(0, 0) and R(x, y) are collinear, then find the relation between x and y.
Given points are P(1, 2), Q(0, 0) and R(x, y).
The given points are collinear, so the area of the triangle formed by them is `square`.
∴ `1/2 |x_1(y_2 - y_3) + x_2(y_3 - y_1) + x_3(y_1 - y_2)| = square`
`1/2 |1(square) + 0(square) + x(square)| = square`
`square + square + square` = 0
`square + square` = 0
`square = square`
Hence, the relation between x and y is `square`.
