Advertisements
Advertisements
प्रश्न
If the points P, Q(x, 7), R, S(6, y) in this order divide the line segment joining A(2, p) and B(7, 10) in 5 equal parts, find x, y and p.
उत्तर
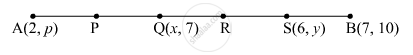
It is given that P, Q(x, 7), R, S(6, y) divides the line segment joining A(2, p) and B(7, 10) in 5 equal parts.
∴ AP = PQ = QR = RS = SB .....(1)
Now,
AP + PQ + QR + RS + SB = AB
⇒ SB + SB + SB + SB + SB = AB [From (1)]
⇒ 5SB = AB
⇒ SB = \[\frac{1}{5}\] AB .....(2)
Now,
AS = AP + PQ + QR + RS = \[\frac{1}{5}\] AB + \[\frac{1}{5}\] AB + \[\frac{1}{5}\] AB + \[\frac{1}{5}\] AB = \[\frac{4}{5}\] AB .....(3)
From (2) and (3), we get
AS : SB = \[\frac{4}{5}\] AB : \[\frac{1}{5}\] AB = 4 : 1
Similarly,
AQ : QB = 2 : 3
Using section formula, we get
Coordinates of Q =
\[ \Rightarrow 20 + 3p = 35\]
\[ \Rightarrow 3p = 15\]
\[ \Rightarrow p = 5\]
Thus, the values of x, y and p are 4, 9 and 5, respectively.
APPEARS IN
संबंधित प्रश्न
How will you describe the position of a table lamp on your study table to another person?
(Street Plan): A city has two main roads which cross each other at the centre of the city. These two roads are along the North-South direction and East-West direction.
All the other streets of the city run parallel to these roads and are 200 m apart. There are 5 streets in each direction. Using 1cm = 200 m, draw a model of the city on your notebook. Represent the roads/streets by single lines.
There are many cross- streets in your model. A particular cross-street is made by two streets, one running in the North - South direction and another in the East - West direction. Each cross street is referred to in the following manner : If the 2nd street running in the North - South direction and 5th in the East - West direction meet at some crossing, then we will call this cross-street (2, 5). Using this convention, find:
- how many cross - streets can be referred to as (4, 3).
- how many cross - streets can be referred to as (3, 4).
Prove that the points (0, 0), (5, 5) and (-5, 5) are the vertices of a right isosceles triangle.
Point A lies on the line segment PQ joining P(6, -6) and Q(-4, -1) in such a way that `(PA)/( PQ)=2/5` . If that point A also lies on the line 3x + k( y + 1 ) = 0, find the value of k.
Points P, Q, R and S divide the line segment joining the points A(1,2) and B(6,7) in five equal parts. Find the coordinates of the points P,Q and R
In what ratio is the line segment joining the points A(-2, -3) and B(3,7) divided by the yaxis? Also, find the coordinates of the point of division.
In what ratio does the line x - y - 2 = 0 divide the line segment joining the points A (3, 1) and B (8, 9)?
Point P(x, 4) lies on the line segment joining the points A(−5, 8) and B(4, −10). Find the ratio in which point P divides the line segment AB. Also find the value of x.
Show that `square` ABCD formed by the vertices A(-4,-7), B(-1,2), C(8,5) and D(5,-4) is a rhombus.
The area of the triangle formed by the points P (0, 1), Q (0, 5) and R (3, 4) is
If A(3, y) is equidistant from points P(8, −3) and Q(7, 6), find the value of y and find the distance AQ.
Show that A (−3, 2), B (−5, −5), C (2,−3), and D (4, 4) are the vertices of a rhombus.
Points P, Q, R and S divides the line segment joining A(1, 2) and B(6, 7) in 5 equal parts. Find the coordinates of the points P, Q and R.
Find the value(s) of k for which the points (3k − 1, k − 2), (k, k − 7) and (k − 1, −k − 2) are collinear.
What is the distance between the points A (c, 0) and B (0, −c)?
If P (x, 6) is the mid-point of the line segment joining A (6, 5) and B (4, y), find y.
The coordinates of the point on X-axis which are equidistant from the points (−3, 4) and (2, 5) are
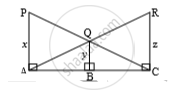
In the above figure, seg PA, seg QB and RC are perpendicular to seg AC. From the information given in the figure, prove that: `1/x + 1/y = 1/z`
Ordinate of all points on the x-axis is ______.
The point at which the two coordinate axes meet is called the ______.
