Question

If the points P, Q(x, 7), R, S(6, y) in this order divide the line segment joining A(2, p) and B(7, 10) in 5 equal parts, find x, y and p. 

Answer 1

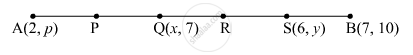
It is given that P, Q(x, 7), R, S(6, y) divides the line segment joining A(2, p) and B(7, 10) in 5 equal parts.

∴ AP = PQ = QR = RS = SB          .....(1)

Now,

AP + PQ + QR + RS + SB = AB

⇒ SB + SB + SB + SB + SB = AB             [From (1)]

⇒ 5SB = AB

⇒ SB = \[\frac{1}{5}\] AB                  .....(2)   
Now,
AS = AP + PQ + QR + RS = \[\frac{1}{5}\] AB + \[\frac{1}{5}\] AB + \[\frac{1}{5}\] AB + \[\frac{1}{5}\] AB = \[\frac{4}{5}\] AB         .....(3)

From (2) and (3), we get
AS : SB =  \[\frac{4}{5}\] AB :  \[\frac{1}{5}\] AB = 4 : 1

Similarly,
AQ : QB = 2 : 3
Using section formula, we get
Coordinates of Q =

\[\left( \frac{2 \times 7 + 3 \times 2}{2 + 3}, \frac{2 \times 10 + 3 \times p}{2 + 3} \right) = \left( \frac{20}{5}, \frac{20 + 3p}{5} \right) = \left( 4, \frac{20 + 3p}{5} \right)\] 

\[\therefore \left( x, 7 \right) = \left( 4, \frac{20 + 3p}{5} \right)\]

\[\Rightarrow x = 4\]   and

\[7 = \frac{20 + 3p}{5}\]

Now,

\[7 = \frac{20 + 3p}{5}\]
\[ \Rightarrow 20 + 3p = 35\]
\[ \Rightarrow 3p = 15\]
\[ \Rightarrow p = 5\]

Coordinates of S = \[\left( \frac{4 \times 7 + 1 \times 2}{4 + 1}, \frac{4 \times 10 + 1 \times p}{4 + 1} \right) = \left( \frac{30}{5}, \frac{40 + 5}{5} \right) = \left( 6, 9 \right)\]

\[\therefore \left( 6, y \right) = \left( 6, 9 \right)\]

\[ \Rightarrow y = 9\]
Thus, the values of x, y and p are 4, 9 and 5, respectively.