Advertisements
Advertisements
प्रश्न
If (x , 2), (−3, −4) and (7, −5) are collinear, then x =
पर्याय
60
63
−63
−60
उत्तर
We have three collinear points A (x , 2 ) ; B ( -3 ,-4) ; C(7 , - 5).
In general if A (x1 , y1) ; B (x2 , y2) ; C (x3 , y 3). are collinear then,
`x_1 ( y_2 -y_3) + x_2 (y_3 - y_1) + x_3 (y_1 - y_2 ) = 0`
So,
x (-4 + 5 ) -3 (-5-2)+ 7 (2 +4) = 0
So,
`x + 42 + 21 = 0`
Therefore,
x = - 63
APPEARS IN
संबंधित प्रश्न
Prove that the points (3, 0), (6, 4) and (-1, 3) are the vertices of a right-angled isosceles triangle.
Which point on the x-axis is equidistant from (5, 9) and (−4, 6)?
Find the centre of the circle passing through (5, -8), (2, -9) and (2, 1).
Find the ratio in which the line segment joining (-2, -3) and (5, 6) is divided by y-axis. Also, find the coordinates of the point of division in each case.
If the poin A(0,2) is equidistant form the points B (3, p) and C (p ,5) find the value of p. Also, find the length of AB.
Show that the points A(2,1), B(5,2), C(6,4) and D(3,3) are the angular points of a parallelogram. Is this figure a rectangle?
Show hat A(1,2), B(4,3),C(6,6) and D(3,5) are the vertices of a parallelogram. Show that ABCD is not rectangle.
Point A lies on the line segment PQ joining P(6, -6) and Q(-4, -1) in such a way that `(PA)/( PQ)=2/5` . If that point A also lies on the line 3x + k( y + 1 ) = 0, find the value of k.
Find the point on x-axis which is equidistant from points A(-1,0) and B(5,0)
Find the coordinates of circumcentre and radius of circumcircle of ∆ABC if A(7, 1), B(3, 5) and C(2, 0) are given.
If (0, −3) and (0, 3) are the two vertices of an equilateral triangle, find the coordinates of its third vertex.
If R (x, y) is a point on the line segment joining the points P (a, b) and Q (b, a), then prove that x + y = a + b.
If three points (x1, y1) (x2, y2), (x3, y3) lie on the same line, prove that \[\frac{y_2 - y_3}{x_2 x_3} + \frac{y_3 - y_1}{x_3 x_1} + \frac{y_1 - y_2}{x_1 x_2} = 0\]
If P is a point on x-axis such that its distance from the origin is 3 units, then the coordinates of a point Q on OY such that OP = OQ, are
Write the X-coordinate and Y-coordinate of point P(– 5, 4)
The line 3x + y – 9 = 0 divides the line joining the points (1, 3) and (2, 7) internally in the ratio ______.
Assertion (A): The ratio in which the line segment joining (2, -3) and (5, 6) internally divided by x-axis is 1:2.
Reason (R): as formula for the internal division is `((mx_2 + nx_1)/(m + n) , (my_2 + ny_1)/(m + n))`
A tiling or tessellation of a flat surface is the covering of a plane using one or more geometric shapes, called tiles, with no overlaps and no gaps. Historically, tessellations were used in ancient Rome and in Islamic art. You may find tessellation patterns on floors, walls, paintings etc. Shown below is a tiled floor in the archaeological Museum of Seville, made using squares, triangles and hexagons.

A craftsman thought of making a floor pattern after being inspired by the above design. To ensure accuracy in his work, he made the pattern on the Cartesian plane. He used regular octagons, squares and triangles for his floor tessellation pattern
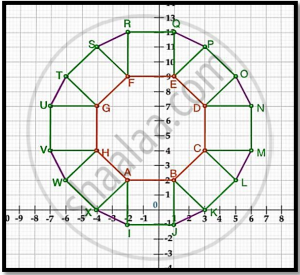
Use the above figure to answer the questions that follow:
- What is the length of the line segment joining points B and F?
- The centre ‘Z’ of the figure will be the point of intersection of the diagonals of quadrilateral WXOP. Then what are the coordinates of Z?
- What are the coordinates of the point on y-axis equidistant from A and G?
OR
What is the area of Trapezium AFGH?
The distance of the point (–6, 8) from x-axis is ______.
Assertion (A): Mid-point of a line segment divides the line segment in the ratio 1 : 1
Reason (R): The ratio in which the point (−3, k) divides the line segment joining the points (− 5, 4) and (− 2, 3) is 1 : 2.
