Advertisements
Advertisements
Question
If `sin theta = a/b` find sec θ + tan θ in terms of a and b.
Advertisements
Solution
We know `sin theta = "𝑜𝑝𝑝𝑜𝑠𝑖𝑡𝑒 𝑠𝑖𝑑𝑒"/"ℎ𝑦𝑝𝑜𝑡𝑒𝑛𝑢𝑠𝑒"`
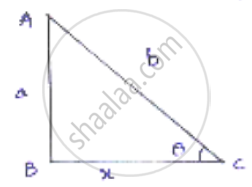
Let x be the adjacent side
By applying Pythagoras theorem
𝐴𝐶2 = 𝐴𝐵2 + 𝐵𝐶2
b2 = a2 + x2
x2 = b2 − a2
`x = sqrt(b^2 - a^2)`
`sec theta = (AB)/(BC) = b/(sqrt(b^2 - a^2))`
`tan theta = (AB)/(BC) = a/(sqrt(b^2 - a^2))`
`sec theta + tan theta = b/(b^2 - a^2) + a/(sqrt(b^2 - a^2))`
`= (b + a)/(sqrt(b^2 - a^2)) = (b+ a)/sqrt((b + a)(b - a)) = (b + a)/sqrt(b + a) - 1/(sqrt(b - a)) = sqrt((b + a)/(b - a))`
APPEARS IN
RELATED QUESTIONS
In ΔABC right angled at B, AB = 24 cm, BC = 7 m. Determine:
sin C, cos C
In Given Figure, find tan P – cot R.
If sin A = `3/4`, calculate cos A and tan A.
If cot θ =` 7/8` evaluate `((1+sin θ )(1-sin θ))/((1+cos θ)(1-cos θ))`
In ΔABC, right angled at B. If tan A = `1/sqrt3` , find the value of
- sin A cos C + cos A sin C
- cos A cos C − sin A sin C
In ΔPQR, right angled at Q, PR + QR = 25 cm and PQ = 5 cm. Determine the values of sin P, cos P and tan P.
In the following, one of the six trigonometric ratios is given. Find the values of the other trigonometric ratios.
`cot theta = 12/5`
If `tan theta = 1/sqrt7` `(cosec^2 theta - sec^2 theta)/(cosec^2 theta + sec^2 theta) = 3/4`
if `sec A = 17/8` verify that `(3 - 4sin^2A)/(4 cos^2 A - 3) = (3 - tan^2 A)/(1 - 3 tan^2 A)`
if `cot theta = 3/4` prove that `sqrt((sec theta - cosec theta)/(sec theta +cosec theta)) = 1/sqrt7`
Evaluate the following
`2 sin^2 30^2 - 3 cos^2 45^2 + tan^2 60^@`
Evaluate the following:
(cosec2 45° sec2 30°)(sin2 30° + 4 cot2 45° − sec2 60°)
Evaluate the Following
cosec3 30° cos 60° tan3 45° sin2 90° sec2 45° cot 30°
Evaluate the Following
(cos 0° + sin 45° + sin 30°)(sin 90° − cos 45° + cos 60°)
Evaluate the Following:
`(tan^2 60^@ + 4 cos^2 45^@ + 3 sec^2 30^@ + 5 cos^2 90)/(cosec 30^@ + sec 60^@ - cot^2 30^@)`
If sec θ = `1/2`, what will be the value of cos θ?
`sqrt(3)` cos2A + `sqrt(3)` sin2A is equal to ______.
Let tan9° = `(1 - sqrt((sqrt(5)k)/m))k` where k = `sqrt(5) + 1` then m is equal to ______.
If sinθ = `1/sqrt(2)` and `π/2 < θ < π`. Then the value of `(sinθ + cosθ)/tanθ` is ______.
