Advertisements
Advertisements
Question
If the height of a cylinder becomes `1/4` of the original height and the radius is doubled, then which of the following will be true?
Options
Total surface area of the cylinder will be doubled.
Total surface area of the cylinder will remain unchanged.
Total surface of the cylinder will be halved.
None of the above.
Solution
None of the above.
Explanation:
We know that,
Total surface area of cylinder having radius r and height h = 2πr (h + r)
Total surface area of the cylinder with new height `(h/u)` and radius 2r
= `2pi(2r) (2r + 1/4 h)`
= `4pir (8r + h) xx 1/4`
= πr (8r + h)
APPEARS IN
RELATED QUESTIONS
The height of a right circular cylinder is 10.5 cm. If three times the sum of the areas of its two circular faces is twice the area of the curved surface area. Find the radius of its base.
In the middle of a rectangular field measuring 30m × 20m, a well of 7 m diameter and 10 m depth is dug. The earth so removed is evenly spread over the remaining part of the field. Find the height through which the level of the field is raised.
The pillars of a temple are cylindrically shaped. If each pillar has a circular base of radius 20 cm and height 10 m. How much concrete mixture would be required to build 14 such pillars?
A cylindrical water tank of diameter 1.4 m and height 2.1 m is being fed by a pipe of diameter 3.5 cm through which water flows at the rate of 2 metre per second. In how much time the tank will be filled?
If the radii of two cylinder are in the ratio 2 : 3 and their heights are in the ratio 5 : 3, then find the ratio of their volumes.
Find the area of base and radius of a cylinder if its curved surface area is 660 sqcm and height is 21 cm.
Find the lateral surface area, total surface area and the volume of the following cylinders: Radius = 4.2cm, Height = 12cm
30 circular plates, each of radius 14 cm and thickness 3 cm are placed one above the another to form a cylindrical solid. Find the total surface area.
In the example given below, the radius of the base of a cylinder and its height is given. Then find the curved surface area and total surface area.
r = 1.4 cm, h = 2.1 cm
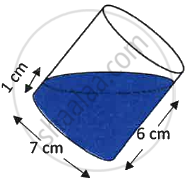
In the adjoining diagram, a tilted right circular cylindrical vessel with base diameter 7 cm contains a liquid. When placed vertically, the height of the liquid in the vessel is the mean of two heights shown in the diagram. Find the area of wet surface, when the cylinder is placed vertically on a horizontal surface. (Use π = `22/7`).